Browse By Unit
Dalia Savy
iliana Polinskiy
Dalia Savy
iliana Polinskiy
SAT Math Overview
Welcome to another SAT Math guide! The math portion of the SAT has four main topics with the Heart of Algebra making up 19/58 questions, or 33% of the math section. To break it down even further, there will be eight Heart of Algebra questions in the non-calculator portion of the math section, and 11 in the calculator portion. They can be either multiple-choice questions or grid-ins!
The Heart of Algebra is an essential part of the SAT that tests your knowledge of analyzing and fluently solving linear equations and inequalities, along with creating systems of equations using various techniques and identifying equations of lines on graphs. You got this! 🍀

Image Courtesy of Tenor
📗Main Heart of Algebra Topic Areas
College Board highlighted a range of skills that might be tested under the category of "Heart of Algebra." Don't worry, we'll put them into four big groups and take you through each of them. For now, here's a checklist of topics pertaining to this part of the math section:
📏 Linear Equations, Linear Inequalities, and Linear Functions in Context
This set of skills is all about representing the context of the given problem algebraically. You'll be defining variables to represent quantities in context, writing out equations, inequalities, or functions, and solving them! The very last component is putting your solution back into context of the real-life situation at hand. 🕵🏻♀️
🔄 Systems of Linear Equations and Inequalities in Context
These skills are all about systems of equations and inequalities, tasking you with creating them and solving them in the context of the problem.
🏋️♂️ Fluency in Solving Linear Equations, Linear Inequalities, and Systems of Linear Equations
Since the second group of skills was all about creating equations and inequalities, this one is about solving them fluently. These questions may not have any context, you would just have to solve the given equation or inequality at hand.
📋 The Relationships among Linear Equations, Lines in the Coordinate Plane, and the Contexts They Describe
This last group of skills will help you visually see a system of equations on a coordinate plane. Once you graph the systems, you'll be able to interpret if the system has one solution, many solutions, or no solution. Woah! 🤯
📏 Linear Equations, Linear Inequalities, and Linear Functions in Context
Let's start out with the first group of skills, centering around representing a real-life situation algebraically.
🧠 What You Need to Know: Linear Equations, Inequalities, and Functions in Context
Now let's get into what you should be able to do for this group:
Identify the difference between linear expressions, equations, and inequalities.
➕ Linear expressions are mathematical phrases that contain a variable ("x") and constants. They won't ever have an equal sign (=) or an inequality symbol (<, >, ≤, ≥). Some examples include 5x-3 or 2y-7.
🟰 Linear equations are mathematical phrases that contain variables, constants, and an equal sign. They express an equality between two linear expressions, such as 5x-3=12 with a solution of x = 3.
Expressions and equations look very similar; the difference is expressions don't have an equal sign.
🤨 Linear inequalities are mathematical statements that contain variables, constants, and inequality symbols (<, >, ≤, ≥). An example is 2x +1 > 5 with a range of solutions x > 2.
Define and assign variables to represent quantities in the question's context.
Formulate expressions, equations, or inequalities based on relationships described in the question.
Solve formulated linear equations and inequalities.
Expressions, equations, and inequalities you work with on the SAT may require multiple steps in order to simplify or solve for the variable.
Linear equations provide us with a single, precise answer such as x=5. On the other hand, linear inequalities offer a range of possible answers where our solution can be located.
Interpret the given or solved solution in the context of the question.
🤓 Applying Your Knowledge: Linear Equations, Inequalities, and Functions in Context
Let's tackle a problem that involves analyzing a real-life situation using linear inequalities. The following question is from the 2020 SAT Study Guide (all credit to College Board).
Interpreting Linear Inequalities Practice
To edit a manuscript, Miguel charges $50 for the first 2 hours and $20 per hour after the first 2 hours. Which of the following expresses the amount, C, in dollars, Miguel charges if it takes him x hours to edit a manuscript, where x > 2?
A) C = 20x
B) C = 20x + 10
C) C = 20x + 50
D) C = 20x + 90
The correct answer is choice B. This is a question involving the interpretation of linear inequalities. Miguel charges $50 for the first two hours, which means there is a flat fee of $50 for the initial 2 hours. After that, he charged $20 per hour.
For the additional hours beyond the first 2 hours, we need to multiply the number of additional hours (x-2) by the rate of $20. When we add these two components together, the correct expression for the amount, C, in dollars is
C = $50 (for the first 2 hours) + ($20 per hour) * (x - 2) (for the additional hours beyond 2)
Simplifying this expression gives us:
C = $50 + $20(x - 2)
C = $50 + $20x - $40
C = $20x + $10
Therefore, the correct expression for the amount, C, in dollars that Miguel charges for x hours of editing a manuscript (where x > 2) is:
C = 20x + 10.
🔄 Systems of Linear Equations and Inequalities in Context
Systems! This set of skills is all about systems of equations and inequalities. Get ready to create some and solve them in the context of the problem.
🧠 What You Need to Know: Systems of Equations and Inequalities in Context
For this group of skills, you should be able to:
Define more than one variable in the context of the problem.
Differentiate between a system of equations and a system of inequalities.
A system of equations is a set of two or more equations that involve multiple variables. These equations must be solved at the same time to find the values of those variables that make every equation in the system true. Essentially, the solution to a system of equations represents the common solution to all the equations in the set.
💡 A system of equations with two variables, x and y, could be 2x+3y=10 and 3x-2y=4.
A system of inequalities is a set of two or more inequalities that involve multiple variables and represent multiple conditions. Unlike a system of equations that has specific solutions, a system of inequalities can be solved to find a range of values that satisfy all the inequalities at the same time.
💡 A system of equations with two variables, x and y, could be 2x+y≤8 and x-3y>2.
Identify when to create a system of equations and when to create a system of inequalities.
A system of equations can be made when you have two or more unknowns that can be related through multiple linear equations, and solving the system will yield a single unique solution. On the other hand, a system of inequalities can be made when you have to find a range of possible solutions for multiple variables.
Formulate a system of equations or inequalities to represent a problem.
🏋️♂️ Fluency in Solving Linear Equations, Linear Inequalities, and Systems of Linear Equations
Next up is getting to solving these equations, inequalities, and systems! Time to become a mathematical master with solving. 🪄
🧠 What You Need to Know: Solving Equations, Inequalities, and Systems
Questions in this skill group can be in either the non-calculator or calculator section, so let's go through some tips for solving linear equations and inequalities. Even the questions in the calculator section can be done by hand to save time! ⏲️
🤔 Tips for Solving Linear Equations
Isolate the Variable: When solving linear equations, you goal is to get the variable alone on one side of the equation.
Perform the Same Operation on Both Sides: Whatever you do to one side of the equation, you must do to the other side to maintain equality.
Combine Like Terms: Simplify the equation as much as possible on each side before isolating the variable. This will make your life easier!
Get Rid of Fractions or Decimals: If the equation you're working with has fractions or decimals, multiply both sides by the least common denominator to get rid of them. It's always easier to work with whole numbers.
Eliminate Wrong Answers: If you're stuck when solving, plug the given answer choices in and see if the equation is true. Plugging in 0 usually helps you eliminate terms. This is a common strategy that we recommend using!
Check Your Solution: Always double-check your work by substituting the answer back into the original equation to make sure both sides are equal. This especially helps with grid-in questions.
💡 Tips for Solving Linear Inequalities
Treat Inequalities like Equations: Most of the tips we went through for linear equations apply to inequalities as well! Remember to isolate the variable, combine like terms, perform the same operation on both sides, and eliminate wrong answers.
Flipping the Sign: If you multiply or divide both sides by a negative number, you must reverse the sign of the inequality.
Get Familiar: Make sure you are familiar with all inequality signs (<, >, ≤, ≥) and maintain the correct sign when simplifying.
Check Solution Range: Always double-check the range of valid solutions for accuracy.
Test for Solutions: To verify the solution's correctness, substitute test points from different regions into the original inequality and observe if they satisfy the inequality.
✨ Tips for Solving Systems of Equations
Choose a Method: There are several ways to solve systems of equations! You can either use elimination or substitution to solve for x and y. Make sure to practice with both approaches so that you know which one will be more convenient on test day!
Using Elimination: Make sure that coefficients are equal and you add or subtract equations to eliminate one of the variables and solve for the other.
Using Substitution: Solve one of the equations for one variable and substitute it into the other equation. Then, you can just solve for the remaining variable.
Identify Special Cases: Be aware of systems with no solution or systems with infinitely many solutions and how to recognize them.
Work Neatly: These can get messy! Keep your work organized and readable to avoid silly mistakes.
😅 Tips for Solving Systems of Inequalities with Absolute Value
Understand Absolute Value Inequalities: The absolute value of a number represents its distance from zero on the number line. Thus, |x| < 3 means the distance of x from zero is less than 3, and |y| > 2 means the distance of y from zero is greater than 2.
Isolate the Absolute Value: Always isolate the absolute value on one side of the inequality.
Solve for Both Cases: Since the absolute value can result in two possible solutions (positive and negative), you have to consider both cases separately.
Identify the Overlapping Region: The overlapping region of the solutions from the two cases will be the final solution to the system of inequalities. This is because both inequalities need to be satisfied simultaneously.
🤓 Applying Your Knowledge: Solving Equations, Inequalities, and Systems
Now, let's solve a linear equation problem to practice! The following question is from the 2020 SAT Study Guide (all credit to College Board).
Solving Linear Equations Practice
What is the solution to the following equation?
−2(3x − 2.4) = −3(3x − 2.4)
Here is how we would go about solving this linear equation:
First, expand the brackets by multiplying the terms inside by the coefficients outside: -6x + 4.8 = -9x + 7.2
Then, move the terms around so all terms with "x" are on one side, and all constants are on the other side: -6x + 9x = -4.8 + 7.2
Simplify both sides and combine like terms: 3x = 2.4
Isolate x by dividing both sides by 3: x = 2.4/3.
Simplify the fraction 2.4/3. This gives you a final answer of x = 0.8.
Therefore, the solution is x = 0.8. This question can be solved without a calculator.
Systems of Inequality with Absolute Value Practice
Which of the following values of x satisfies both inequalities |x - 1| < 3 and |x + 2| > 4?
A) x= 3
B) x = -6
C) x = -4
D) x =-3
The answer is A.
To solve a system of inequalities involving absolute value, we can rewrite each inequality as two inequalities without absolute value and then find where they overlap. For example, |x - 1| < 3 can be rewritten as -3 < x - 1 < 3, which means x must be between -2 and 4 when added by 1. Similarly, |x + 2| > 4 can be rewritten as x + 2 > 4 or x + 2 < -4, which means x must be greater than 2 or less than -6 when subtracted by 2. Subtracting 2 from all sides, we get x > 2 or x < -6. The only value of x that satisfies both inequalities is 3, which is option A.
Great job! Let's move on to the last set of skills. 🥳
📋 The Relationships among Linear Equations, Lines in the Coordinate Plane, and the Contexts They Describe
Last but not least, let's get to graphing! Now that we can handle systems of equations algebraically, let's see what they look like visually on a coordinate plane.
🧠 What You Need to Know: Graphing Linear Equations and Systems
Not only can you use elimination and substitution to solve a system of equations, but you can also graph it on a coordinate plane and find its solutions.
Graphing Systems of Equations
To get you comfortable with graphing, here is a little checklist you can follow:
Write Equations in Slope-Intercept Form: If the equation is not already in slope-intercept form (y = mx + b), rearrange it to get it in this form. This form makes it easier to identify the slope and y-intercept.
Identify the Slope and Y-Intercept: From the equation, determine the slope (m) and the y-intercept (b).
Plot the Y-Intercept: Start by plotting the y-intercept on the y-axis at the point (0, b).
Use the Slope to Plot: Use the slope to plot the rest of the line. From the y-intercept, move up or down the slope and right or left the corresponding number of units. Then, draw a straight line through the points!
Once you have the equations graphed, you can take a look at which of the three possibilities the system fits into:
✅ One Unique Solution: If you see the lines intersecting at one point, the system has a unique solution.
❌ No Solution: If the lines are parallel, they never cross, so the system has no solution.
♾️ Infinite Solutions: If the lines are identical (woah!), every point on the lines is a solution, creating infinite solutions.
You may be wondering how you're going to graph on the SAT with limited time. Well, if you have a graphing calculator and you are working on the calculator section, you can use this method. Otherwise, you can analyze the equations in slope-intercept form to figure out solutions. ⤵️
Analyzing Systems of Equations
Back to algebra, we go! Once you have the equations in slope-intercept form, you can use these rules:
If the two lines have the same slope (m) and different y-intercepts (b), they are parallel and the system has no solution.
If the two lines have the same slope (m) and the same y-intercept (b), they are identical and the system has infinite solutions.
A couple of other things to note:
If you have any two points or a single point plus b, you can solve for slope.
When solving for slope when you are given two points keep this formula in mind:
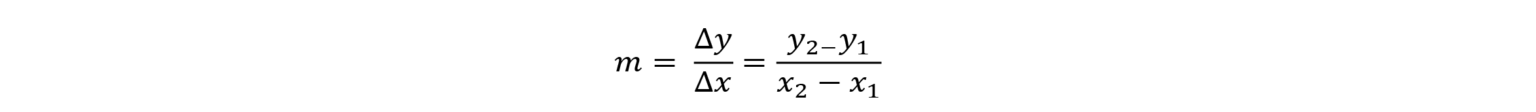
Image Courtesy of Test Geek
If you know the slope, y-intercept, and y, you can solve for x. Vice versa is true as well; if you know the slope, y-intercept, and x, you can solve for y.
To do this, we would use the point-slope form of a linear equation: y - y₁ = m(x - x₁), where (x₁, y₁) denotes the coordinates of the given point on the line, and 'm' represents the slope.
When two lines, A and B, are perpendicular to each other, they intersect at a right angle. This means that the slope of line A will be the negative reciprocal of the slope of line B (ma = -1/mb).
Graphing Inequalities
Before we move on, let's cover some steps for graphing inequalities:
Treat it like an Equation. First, you should treat the inequality as an equation and follow the steps that we outlined before.
Determine the shading direction. If the inequality is greater than (>), the shaded region should be above the graphed line. If the inequality is less than (<), the shaded region should be below the line. Shade the appropriate region.
Check if the boundary line is included. If the inequality includes ≥ or ≤, use a solid line for the boundary. Otherwise, use a dashed line to indicate that the line itself is not included in the solution.
🤓 Applying Your Knowledge: Graphing Linear Equations and Systems
Solving Systems of Equations Algebraically and Graphically Practice
How many Solutions (x,y) does the given system of equations have?
2y+6x=3
y+3x=2
A) Zero
B) Exactly one
C) Exactly two
D) Infinitely many
The correct answer is A.
Solution & Advice:
To determine the number of solutions that satisfy both equations, we can analyze the relationship between the two equations. One way you can solve this problem is to graph the two equations. In this case, we have a situation where the lines represented by these equations are parallel. When two lines are parallel, they never intersect, meaning they don't share a common solution. That is why zero values of (x, y) satisfy both equations simultaneously.
You can also solve this problem algebraically! If you multiply each side of the equation y + 3x=2 by 2 you get 2y+6x=4. Then, subtracting each side of 2y+ 6x = 3 from the corresponding side of 2y + 6x=4 gives 0=1. Since 0=1 is a false statement, the system has zero solutions.
Writing the Equation of a Line: Point-Slope Form Practice
Which of the following is the correct way to write the equation of a line that passes through the point (2, 3) and has a slope of 2?
A) y= 2x-1
B) y= 2x+1
C) y = -2x+5
D) y= -2x-5
The correct answer is A.
Solution & Advice:
To write the equation of a line that passes through a given point (x₁, y₁) and has a given slope 'm,' we use the point-slope form of a linear equation. This form is represented as y - y₁ = m(x - x₁), where (x₁, y₁) denotes the coordinates of the given point on the line, and 'm' represents the slope.
Here, we first have to identify the point (x₁, y₁) through which the line passes and the slope (m), which are (2, 3) and 4 respectively. Then, we substitute x₁ = 2, y₁ = 3, and m = 2 into the point-slope form: y - 3 = 2(x - 2). Now, we simplify the equation by performing operations, giving us y - 3 = 2x - 2. If required, we can further convert this to slope-intercept form (y = mx + b) by isolating 'y' on one side, which in this case would be y = 2x - 1. Always verify the result by ensuring that the equation represents a line with the correct slope and passes through the given point.
Graphing Inequalities Practice
Which of the following graphs represents the solution to the inequality x + 2y < 4?
First, treat the inequality as an equation (x + 2y = 4) and graph the boundary line that represents it.
To graph the line, first, find two points that lie on it. Choose x = 0 and solve for y: 0 + 2y = 4 => y = 2, giving us one point (0, 2). Then choose y = 0 and solve for x: x + 2(0) = 4 => x = 4, giving us the second point (4, 0).
Plot these points on the coordinate plane and draw a straight line passing through them. The boundary line should be a dashed line since the original inequality is < (less than), not ≤ (less than or equal to).
Next, determine which side of the boundary line to shade to represent the solution to the inequality.
Since the inequality is x + 2y < 4, the shaded region should be below the boundary line. To check which side is below, choose a point not on the line, like (0, 0), and substitute it into the inequality: 0 + 2(0) < 4, which is true.
Shade the region below the dashed line to indicate the solution set.
Finally, check if the boundary line should be included in the solution. Since the original inequality is < (less than) and not ≤ (less than or equal to), the boundary line is not included. Therefore, the line should remain dashed in the graph.
Double-check that you have correctly graphed the solution by testing a point from the shaded region and a point from outside the shaded region. Both points should satisfy the original inequality.
The graph representing the solution to the inequality x + 2y < 4 is a dashed boundary line passing through (0, 2) and (4, 0), with the shaded region below the line, and the line is not included in the solution. Make sure to practice similar problems to gain confidence in graphing inequalities accurately. Good luck!
⭐️ Closing
Congratulations! You have made it to the end of this SAT Math-Heart of Algebra. 👏 Good luck studying for the SAT Math section!
Need more SAT resources? Check out our SAT Math Section Tips and Tricks. Want to see more practice questions. Take a look at "What are the SAT Math Test Questions Like?"
Keep up the great work!! 🥳
<< Hide Menu
Dalia Savy
iliana Polinskiy
Dalia Savy
iliana Polinskiy
SAT Math Overview
Welcome to another SAT Math guide! The math portion of the SAT has four main topics with the Heart of Algebra making up 19/58 questions, or 33% of the math section. To break it down even further, there will be eight Heart of Algebra questions in the non-calculator portion of the math section, and 11 in the calculator portion. They can be either multiple-choice questions or grid-ins!
The Heart of Algebra is an essential part of the SAT that tests your knowledge of analyzing and fluently solving linear equations and inequalities, along with creating systems of equations using various techniques and identifying equations of lines on graphs. You got this! 🍀

Image Courtesy of Tenor
📗Main Heart of Algebra Topic Areas
College Board highlighted a range of skills that might be tested under the category of "Heart of Algebra." Don't worry, we'll put them into four big groups and take you through each of them. For now, here's a checklist of topics pertaining to this part of the math section:
📏 Linear Equations, Linear Inequalities, and Linear Functions in Context
This set of skills is all about representing the context of the given problem algebraically. You'll be defining variables to represent quantities in context, writing out equations, inequalities, or functions, and solving them! The very last component is putting your solution back into context of the real-life situation at hand. 🕵🏻♀️
🔄 Systems of Linear Equations and Inequalities in Context
These skills are all about systems of equations and inequalities, tasking you with creating them and solving them in the context of the problem.
🏋️♂️ Fluency in Solving Linear Equations, Linear Inequalities, and Systems of Linear Equations
Since the second group of skills was all about creating equations and inequalities, this one is about solving them fluently. These questions may not have any context, you would just have to solve the given equation or inequality at hand.
📋 The Relationships among Linear Equations, Lines in the Coordinate Plane, and the Contexts They Describe
This last group of skills will help you visually see a system of equations on a coordinate plane. Once you graph the systems, you'll be able to interpret if the system has one solution, many solutions, or no solution. Woah! 🤯
📏 Linear Equations, Linear Inequalities, and Linear Functions in Context
Let's start out with the first group of skills, centering around representing a real-life situation algebraically.
🧠 What You Need to Know: Linear Equations, Inequalities, and Functions in Context
Now let's get into what you should be able to do for this group:
Identify the difference between linear expressions, equations, and inequalities.
➕ Linear expressions are mathematical phrases that contain a variable ("x") and constants. They won't ever have an equal sign (=) or an inequality symbol (<, >, ≤, ≥). Some examples include 5x-3 or 2y-7.
🟰 Linear equations are mathematical phrases that contain variables, constants, and an equal sign. They express an equality between two linear expressions, such as 5x-3=12 with a solution of x = 3.
Expressions and equations look very similar; the difference is expressions don't have an equal sign.
🤨 Linear inequalities are mathematical statements that contain variables, constants, and inequality symbols (<, >, ≤, ≥). An example is 2x +1 > 5 with a range of solutions x > 2.
Define and assign variables to represent quantities in the question's context.
Formulate expressions, equations, or inequalities based on relationships described in the question.
Solve formulated linear equations and inequalities.
Expressions, equations, and inequalities you work with on the SAT may require multiple steps in order to simplify or solve for the variable.
Linear equations provide us with a single, precise answer such as x=5. On the other hand, linear inequalities offer a range of possible answers where our solution can be located.
Interpret the given or solved solution in the context of the question.
🤓 Applying Your Knowledge: Linear Equations, Inequalities, and Functions in Context
Let's tackle a problem that involves analyzing a real-life situation using linear inequalities. The following question is from the 2020 SAT Study Guide (all credit to College Board).
Interpreting Linear Inequalities Practice
To edit a manuscript, Miguel charges $50 for the first 2 hours and $20 per hour after the first 2 hours. Which of the following expresses the amount, C, in dollars, Miguel charges if it takes him x hours to edit a manuscript, where x > 2?
A) C = 20x
B) C = 20x + 10
C) C = 20x + 50
D) C = 20x + 90
The correct answer is choice B. This is a question involving the interpretation of linear inequalities. Miguel charges $50 for the first two hours, which means there is a flat fee of $50 for the initial 2 hours. After that, he charged $20 per hour.
For the additional hours beyond the first 2 hours, we need to multiply the number of additional hours (x-2) by the rate of $20. When we add these two components together, the correct expression for the amount, C, in dollars is
C = $50 (for the first 2 hours) + ($20 per hour) * (x - 2) (for the additional hours beyond 2)
Simplifying this expression gives us:
C = $50 + $20(x - 2)
C = $50 + $20x - $40
C = $20x + $10
Therefore, the correct expression for the amount, C, in dollars that Miguel charges for x hours of editing a manuscript (where x > 2) is:
C = 20x + 10.
🔄 Systems of Linear Equations and Inequalities in Context
Systems! This set of skills is all about systems of equations and inequalities. Get ready to create some and solve them in the context of the problem.
🧠 What You Need to Know: Systems of Equations and Inequalities in Context
For this group of skills, you should be able to:
Define more than one variable in the context of the problem.
Differentiate between a system of equations and a system of inequalities.
A system of equations is a set of two or more equations that involve multiple variables. These equations must be solved at the same time to find the values of those variables that make every equation in the system true. Essentially, the solution to a system of equations represents the common solution to all the equations in the set.
💡 A system of equations with two variables, x and y, could be 2x+3y=10 and 3x-2y=4.
A system of inequalities is a set of two or more inequalities that involve multiple variables and represent multiple conditions. Unlike a system of equations that has specific solutions, a system of inequalities can be solved to find a range of values that satisfy all the inequalities at the same time.
💡 A system of equations with two variables, x and y, could be 2x+y≤8 and x-3y>2.
Identify when to create a system of equations and when to create a system of inequalities.
A system of equations can be made when you have two or more unknowns that can be related through multiple linear equations, and solving the system will yield a single unique solution. On the other hand, a system of inequalities can be made when you have to find a range of possible solutions for multiple variables.
Formulate a system of equations or inequalities to represent a problem.
🏋️♂️ Fluency in Solving Linear Equations, Linear Inequalities, and Systems of Linear Equations
Next up is getting to solving these equations, inequalities, and systems! Time to become a mathematical master with solving. 🪄
🧠 What You Need to Know: Solving Equations, Inequalities, and Systems
Questions in this skill group can be in either the non-calculator or calculator section, so let's go through some tips for solving linear equations and inequalities. Even the questions in the calculator section can be done by hand to save time! ⏲️
🤔 Tips for Solving Linear Equations
Isolate the Variable: When solving linear equations, you goal is to get the variable alone on one side of the equation.
Perform the Same Operation on Both Sides: Whatever you do to one side of the equation, you must do to the other side to maintain equality.
Combine Like Terms: Simplify the equation as much as possible on each side before isolating the variable. This will make your life easier!
Get Rid of Fractions or Decimals: If the equation you're working with has fractions or decimals, multiply both sides by the least common denominator to get rid of them. It's always easier to work with whole numbers.
Eliminate Wrong Answers: If you're stuck when solving, plug the given answer choices in and see if the equation is true. Plugging in 0 usually helps you eliminate terms. This is a common strategy that we recommend using!
Check Your Solution: Always double-check your work by substituting the answer back into the original equation to make sure both sides are equal. This especially helps with grid-in questions.
💡 Tips for Solving Linear Inequalities
Treat Inequalities like Equations: Most of the tips we went through for linear equations apply to inequalities as well! Remember to isolate the variable, combine like terms, perform the same operation on both sides, and eliminate wrong answers.
Flipping the Sign: If you multiply or divide both sides by a negative number, you must reverse the sign of the inequality.
Get Familiar: Make sure you are familiar with all inequality signs (<, >, ≤, ≥) and maintain the correct sign when simplifying.
Check Solution Range: Always double-check the range of valid solutions for accuracy.
Test for Solutions: To verify the solution's correctness, substitute test points from different regions into the original inequality and observe if they satisfy the inequality.
✨ Tips for Solving Systems of Equations
Choose a Method: There are several ways to solve systems of equations! You can either use elimination or substitution to solve for x and y. Make sure to practice with both approaches so that you know which one will be more convenient on test day!
Using Elimination: Make sure that coefficients are equal and you add or subtract equations to eliminate one of the variables and solve for the other.
Using Substitution: Solve one of the equations for one variable and substitute it into the other equation. Then, you can just solve for the remaining variable.
Identify Special Cases: Be aware of systems with no solution or systems with infinitely many solutions and how to recognize them.
Work Neatly: These can get messy! Keep your work organized and readable to avoid silly mistakes.
😅 Tips for Solving Systems of Inequalities with Absolute Value
Understand Absolute Value Inequalities: The absolute value of a number represents its distance from zero on the number line. Thus, |x| < 3 means the distance of x from zero is less than 3, and |y| > 2 means the distance of y from zero is greater than 2.
Isolate the Absolute Value: Always isolate the absolute value on one side of the inequality.
Solve for Both Cases: Since the absolute value can result in two possible solutions (positive and negative), you have to consider both cases separately.
Identify the Overlapping Region: The overlapping region of the solutions from the two cases will be the final solution to the system of inequalities. This is because both inequalities need to be satisfied simultaneously.
🤓 Applying Your Knowledge: Solving Equations, Inequalities, and Systems
Now, let's solve a linear equation problem to practice! The following question is from the 2020 SAT Study Guide (all credit to College Board).
Solving Linear Equations Practice
What is the solution to the following equation?
−2(3x − 2.4) = −3(3x − 2.4)
Here is how we would go about solving this linear equation:
First, expand the brackets by multiplying the terms inside by the coefficients outside: -6x + 4.8 = -9x + 7.2
Then, move the terms around so all terms with "x" are on one side, and all constants are on the other side: -6x + 9x = -4.8 + 7.2
Simplify both sides and combine like terms: 3x = 2.4
Isolate x by dividing both sides by 3: x = 2.4/3.
Simplify the fraction 2.4/3. This gives you a final answer of x = 0.8.
Therefore, the solution is x = 0.8. This question can be solved without a calculator.
Systems of Inequality with Absolute Value Practice
Which of the following values of x satisfies both inequalities |x - 1| < 3 and |x + 2| > 4?
A) x= 3
B) x = -6
C) x = -4
D) x =-3
The answer is A.
To solve a system of inequalities involving absolute value, we can rewrite each inequality as two inequalities without absolute value and then find where they overlap. For example, |x - 1| < 3 can be rewritten as -3 < x - 1 < 3, which means x must be between -2 and 4 when added by 1. Similarly, |x + 2| > 4 can be rewritten as x + 2 > 4 or x + 2 < -4, which means x must be greater than 2 or less than -6 when subtracted by 2. Subtracting 2 from all sides, we get x > 2 or x < -6. The only value of x that satisfies both inequalities is 3, which is option A.
Great job! Let's move on to the last set of skills. 🥳
📋 The Relationships among Linear Equations, Lines in the Coordinate Plane, and the Contexts They Describe
Last but not least, let's get to graphing! Now that we can handle systems of equations algebraically, let's see what they look like visually on a coordinate plane.
🧠 What You Need to Know: Graphing Linear Equations and Systems
Not only can you use elimination and substitution to solve a system of equations, but you can also graph it on a coordinate plane and find its solutions.
Graphing Systems of Equations
To get you comfortable with graphing, here is a little checklist you can follow:
Write Equations in Slope-Intercept Form: If the equation is not already in slope-intercept form (y = mx + b), rearrange it to get it in this form. This form makes it easier to identify the slope and y-intercept.
Identify the Slope and Y-Intercept: From the equation, determine the slope (m) and the y-intercept (b).
Plot the Y-Intercept: Start by plotting the y-intercept on the y-axis at the point (0, b).
Use the Slope to Plot: Use the slope to plot the rest of the line. From the y-intercept, move up or down the slope and right or left the corresponding number of units. Then, draw a straight line through the points!
Once you have the equations graphed, you can take a look at which of the three possibilities the system fits into:
✅ One Unique Solution: If you see the lines intersecting at one point, the system has a unique solution.
❌ No Solution: If the lines are parallel, they never cross, so the system has no solution.
♾️ Infinite Solutions: If the lines are identical (woah!), every point on the lines is a solution, creating infinite solutions.
You may be wondering how you're going to graph on the SAT with limited time. Well, if you have a graphing calculator and you are working on the calculator section, you can use this method. Otherwise, you can analyze the equations in slope-intercept form to figure out solutions. ⤵️
Analyzing Systems of Equations
Back to algebra, we go! Once you have the equations in slope-intercept form, you can use these rules:
If the two lines have the same slope (m) and different y-intercepts (b), they are parallel and the system has no solution.
If the two lines have the same slope (m) and the same y-intercept (b), they are identical and the system has infinite solutions.
A couple of other things to note:
If you have any two points or a single point plus b, you can solve for slope.
When solving for slope when you are given two points keep this formula in mind:
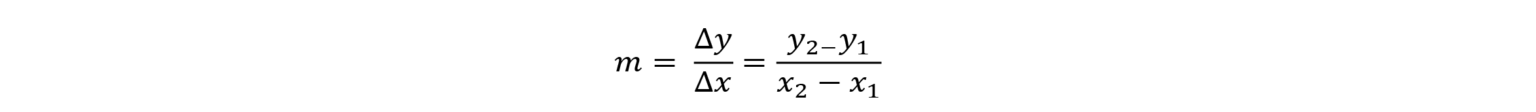
Image Courtesy of Test Geek
If you know the slope, y-intercept, and y, you can solve for x. Vice versa is true as well; if you know the slope, y-intercept, and x, you can solve for y.
To do this, we would use the point-slope form of a linear equation: y - y₁ = m(x - x₁), where (x₁, y₁) denotes the coordinates of the given point on the line, and 'm' represents the slope.
When two lines, A and B, are perpendicular to each other, they intersect at a right angle. This means that the slope of line A will be the negative reciprocal of the slope of line B (ma = -1/mb).
Graphing Inequalities
Before we move on, let's cover some steps for graphing inequalities:
Treat it like an Equation. First, you should treat the inequality as an equation and follow the steps that we outlined before.
Determine the shading direction. If the inequality is greater than (>), the shaded region should be above the graphed line. If the inequality is less than (<), the shaded region should be below the line. Shade the appropriate region.
Check if the boundary line is included. If the inequality includes ≥ or ≤, use a solid line for the boundary. Otherwise, use a dashed line to indicate that the line itself is not included in the solution.
🤓 Applying Your Knowledge: Graphing Linear Equations and Systems
Solving Systems of Equations Algebraically and Graphically Practice
How many Solutions (x,y) does the given system of equations have?
2y+6x=3
y+3x=2
A) Zero
B) Exactly one
C) Exactly two
D) Infinitely many
The correct answer is A.
Solution & Advice:
To determine the number of solutions that satisfy both equations, we can analyze the relationship between the two equations. One way you can solve this problem is to graph the two equations. In this case, we have a situation where the lines represented by these equations are parallel. When two lines are parallel, they never intersect, meaning they don't share a common solution. That is why zero values of (x, y) satisfy both equations simultaneously.
You can also solve this problem algebraically! If you multiply each side of the equation y + 3x=2 by 2 you get 2y+6x=4. Then, subtracting each side of 2y+ 6x = 3 from the corresponding side of 2y + 6x=4 gives 0=1. Since 0=1 is a false statement, the system has zero solutions.
Writing the Equation of a Line: Point-Slope Form Practice
Which of the following is the correct way to write the equation of a line that passes through the point (2, 3) and has a slope of 2?
A) y= 2x-1
B) y= 2x+1
C) y = -2x+5
D) y= -2x-5
The correct answer is A.
Solution & Advice:
To write the equation of a line that passes through a given point (x₁, y₁) and has a given slope 'm,' we use the point-slope form of a linear equation. This form is represented as y - y₁ = m(x - x₁), where (x₁, y₁) denotes the coordinates of the given point on the line, and 'm' represents the slope.
Here, we first have to identify the point (x₁, y₁) through which the line passes and the slope (m), which are (2, 3) and 4 respectively. Then, we substitute x₁ = 2, y₁ = 3, and m = 2 into the point-slope form: y - 3 = 2(x - 2). Now, we simplify the equation by performing operations, giving us y - 3 = 2x - 2. If required, we can further convert this to slope-intercept form (y = mx + b) by isolating 'y' on one side, which in this case would be y = 2x - 1. Always verify the result by ensuring that the equation represents a line with the correct slope and passes through the given point.
Graphing Inequalities Practice
Which of the following graphs represents the solution to the inequality x + 2y < 4?
First, treat the inequality as an equation (x + 2y = 4) and graph the boundary line that represents it.
To graph the line, first, find two points that lie on it. Choose x = 0 and solve for y: 0 + 2y = 4 => y = 2, giving us one point (0, 2). Then choose y = 0 and solve for x: x + 2(0) = 4 => x = 4, giving us the second point (4, 0).
Plot these points on the coordinate plane and draw a straight line passing through them. The boundary line should be a dashed line since the original inequality is < (less than), not ≤ (less than or equal to).
Next, determine which side of the boundary line to shade to represent the solution to the inequality.
Since the inequality is x + 2y < 4, the shaded region should be below the boundary line. To check which side is below, choose a point not on the line, like (0, 0), and substitute it into the inequality: 0 + 2(0) < 4, which is true.
Shade the region below the dashed line to indicate the solution set.
Finally, check if the boundary line should be included in the solution. Since the original inequality is < (less than) and not ≤ (less than or equal to), the boundary line is not included. Therefore, the line should remain dashed in the graph.
Double-check that you have correctly graphed the solution by testing a point from the shaded region and a point from outside the shaded region. Both points should satisfy the original inequality.
The graph representing the solution to the inequality x + 2y < 4 is a dashed boundary line passing through (0, 2) and (4, 0), with the shaded region below the line, and the line is not included in the solution. Make sure to practice similar problems to gain confidence in graphing inequalities accurately. Good luck!
⭐️ Closing
Congratulations! You have made it to the end of this SAT Math-Heart of Algebra. 👏 Good luck studying for the SAT Math section!
Need more SAT resources? Check out our SAT Math Section Tips and Tricks. Want to see more practice questions. Take a look at "What are the SAT Math Test Questions Like?"
Keep up the great work!! 🥳

© 2024 Fiveable Inc. All rights reserved.