Browse By Unit
7.4 Reasoning Using Slope Fields
In the last guide, we learned how to construct slope fields from differential equations. But why are slope fields useful? What information can we glean from them? In this guide, we’ll explore how to find critical points using slope fields and how solutions to slope fields are actually families of functions. 🤯
🤔 What is a Slope Field?
Before diving into the applications, let’s review what a slope field is. Slope fields, also known as direction fields, offer a visual approach to understanding the solutions of differential equations. These graphical representations provide a snapshot of how a function's slope changes at different points in the coordinate plane.
Below is a grid superimposed on the coordinate plane, with each grid point featuring a tiny line segment. This line segment serves as a mini-arrow, showing us the slope of a potential solution at that particular point. The direction of the line segment indicates the direction in which the function is changing (positive, no change, or negative), while the steepness represents the magnitude of the slope.
This visual helps you anticipate the behavior of solutions to differential equations without solving them explicitly! 🖌️
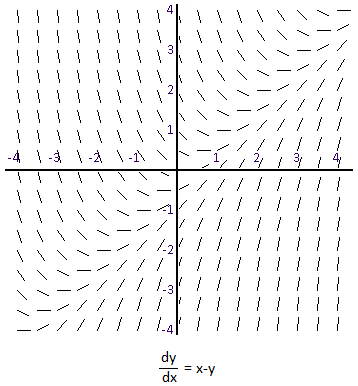
Image courtesy of Coping with Calculus.
🧠 Extracting Information from Slope Fields
Each segment encapsulates a specific slope, a numerical representation of the rate of change at that precise location. The way these lines point is like a guide, showing us which way the function is going. By looking at how steep and which way these lines are leaning, we can learn a lot about what the function is doing, like finding patterns and trends hidden in the differential equation. 👀
🎯 Using Slope Fields to Find Critical Points
Since slope fields map out differential equations, which are the derivatives of functions, we can use them to find the critical points—where the slope of a function is zero or undefined—of a particular solution.
To do this, we first need to identify regions where the line segments are horizontal and flat, indicating a slope of zero. These regions are potential candidates for critical points.
For example, in the slope field above for the differential equation , there are horizontal line segments along the line , meaning the slope is zero at those points. In the differential equation, when x and y are the same, subtracting them yields a difference of zero, so those points are candidates for critical points.
Critical points also occur when the slope is undefined, which is represented by a vertical line in a slope field. In our example, there are lines that almost look vertical, but none are perfectly straight up, so there are no undefined slopes in this case.
🏁 Solutions as Functions or Families of Functions
When solving differential equations, the introduction of the constant of integration, often denoted as "," is a key element that gives rise to a family of functions. The constant of integration represents an arbitrary constant value that gets added during the integration process. Why is this important? It's because it leads to not just one solution, but a whole group of possible solutions, which we call a family of functions. 👪
Finding the solution of a differential equation requires us to find the indefinite integral of the equation, which includes the constant of integration. This constant accounts for the fact that the derivative of a constant is always zero, and when integrating, we lose information about the specific value of the constant. Its inclusion acknowledges that there are numerous possibilities for the specific function that satisfies the differential equation. Essentially, the constant of integration allows for a wide range of solutions, each differing by the value of this constant.
The introduction of the arbitrary constant leads to a family of functions because, for each unique value of "+C," we obtain a distinct solution to the differential equation. The family encompasses all possible solutions, forming a spectrum of functions with varying characteristics. To pinpoint a particular solution from the family, we often need additional information or initial conditions. These conditions provide specific values that, when substituted into the general solution with the "+C," uniquely determine the constant and, consequently, a particular function within the family. Below is a graph containing the family of solution curves to a differential equation. Each curve on its own is a particular solution with a specific constant in place of “+C” and together the curves constitute the family of functions that are solutions to the differential equation.
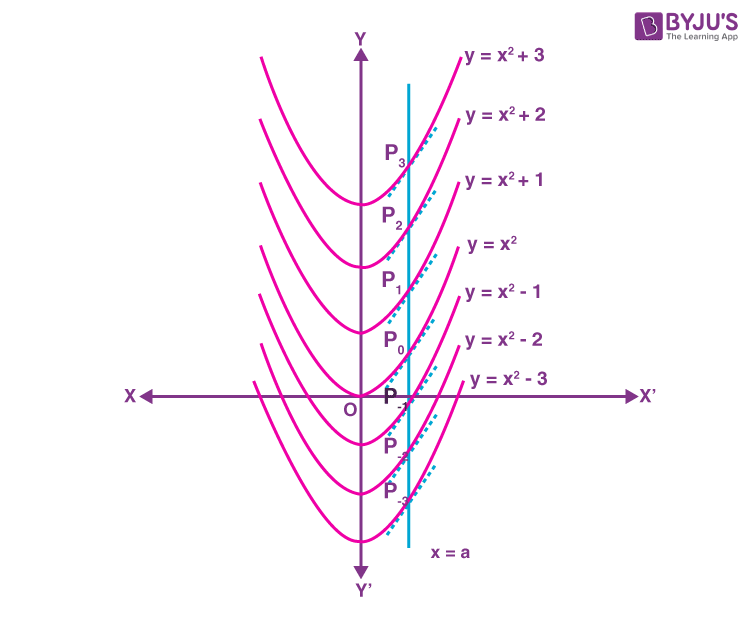
Image courtesy of BYJU’s.
Each curve contains the same parent function, but each curve is slightly different due to “+C” being replaced by -1, -2, -3, and so on.
✏️ Slope Fields Practice
Try applying some of this information below!
🎯 Critical Points
Given the slope field below, find all possible critical points of the original function.
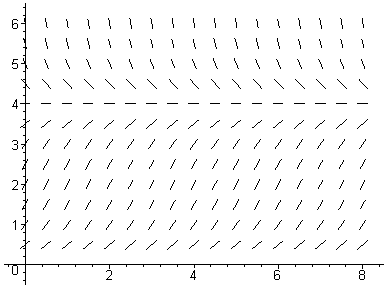
Image courtesy of PBworks.
Recall that critical points occur when the slope of a function is zero or undefined. Since a differential equation models the slope of a function, we simply need to find when there are horizontal line segments in the slope field where the slope would be zero, and when there are vertical line segments that show the slope at that point is undefined.
In the slope field above, we can see that along the line , there are horizontal line segments, indicating the slope is zero. Upon further examination, some of the lines come close to being vertical, but there are no vertical lines present in the slope field.
From this information, we can conclude that possible critical points lie along the line .
🏁 Family of Functions
Let’s solve the differential equation and graph its solutions to see how a family of functions is created.
This differential equation is the derivative of . If you didn’t know that, be sure to review your trig derivatives and inverse trig derivatives! As always when taking a definite integral, we have to include a “+C” to represent the constant of integration because this differential equation could be the derivative of , , or any value of C. So, our solution to this differential equation becomes .
Since C could be any real number, let’s graph our solution curve with different values of C to create a family of functions.
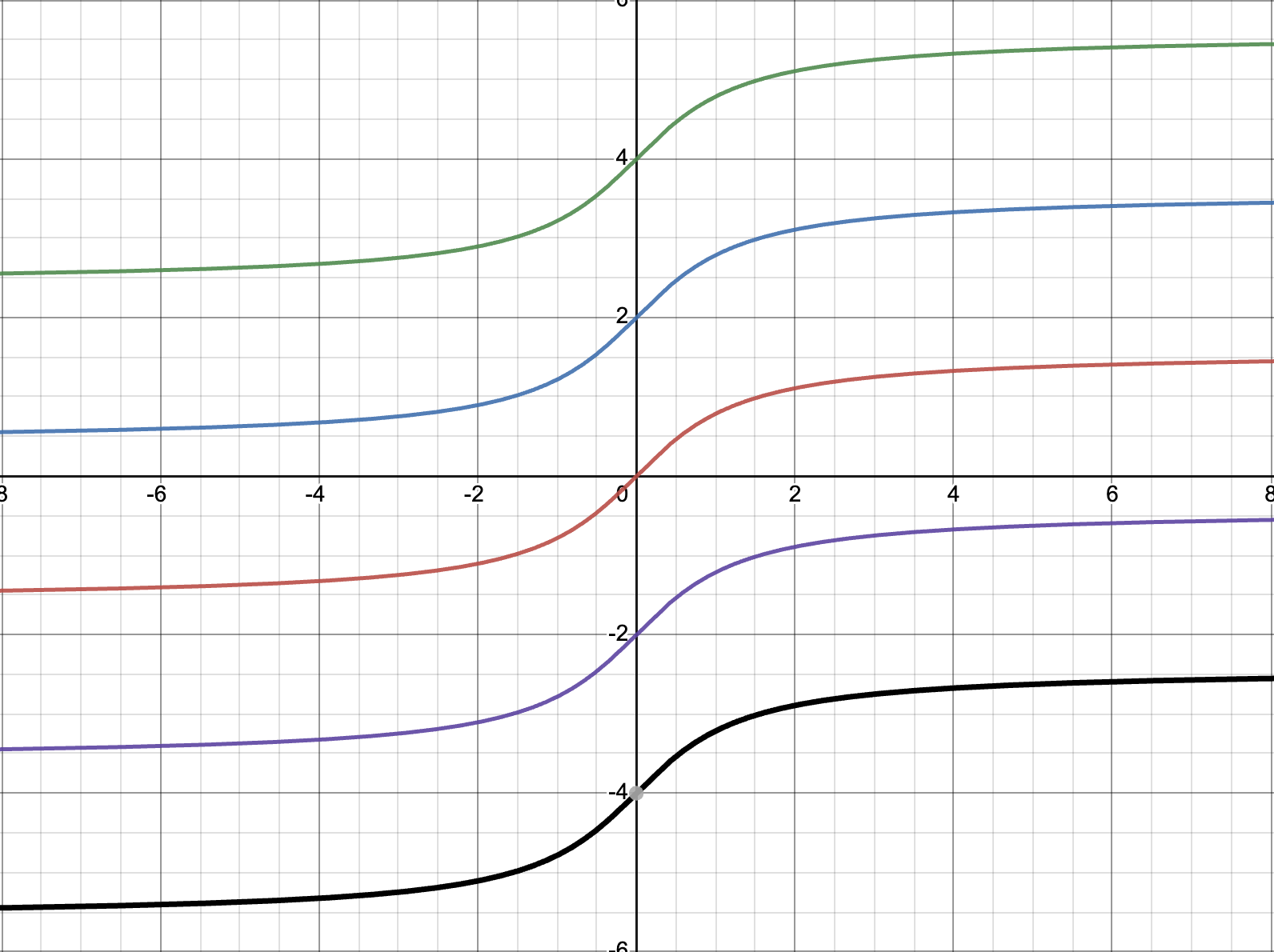
Image courtesy of Desmos.
As you can see, the solution to the differential curve could be any one of the above functions since we were not given an initial value that would allow us to find a particular solution.
✨ Closing
To sum it up, we've covered slope fields and how they play a role in understanding differential equations visually. We also explored how solving differential equations introduces the "+C" constant, leading to a family of functions. Each solution in the family is a bit different due to this constant. We emphasized the need for extra info or initial conditions to pin down a specific solution within the family. 📚
<< Hide Menu
7.4 Reasoning Using Slope Fields
In the last guide, we learned how to construct slope fields from differential equations. But why are slope fields useful? What information can we glean from them? In this guide, we’ll explore how to find critical points using slope fields and how solutions to slope fields are actually families of functions. 🤯
🤔 What is a Slope Field?
Before diving into the applications, let’s review what a slope field is. Slope fields, also known as direction fields, offer a visual approach to understanding the solutions of differential equations. These graphical representations provide a snapshot of how a function's slope changes at different points in the coordinate plane.
Below is a grid superimposed on the coordinate plane, with each grid point featuring a tiny line segment. This line segment serves as a mini-arrow, showing us the slope of a potential solution at that particular point. The direction of the line segment indicates the direction in which the function is changing (positive, no change, or negative), while the steepness represents the magnitude of the slope.
This visual helps you anticipate the behavior of solutions to differential equations without solving them explicitly! 🖌️

Image courtesy of Coping with Calculus.
🧠 Extracting Information from Slope Fields
Each segment encapsulates a specific slope, a numerical representation of the rate of change at that precise location. The way these lines point is like a guide, showing us which way the function is going. By looking at how steep and which way these lines are leaning, we can learn a lot about what the function is doing, like finding patterns and trends hidden in the differential equation. 👀
🎯 Using Slope Fields to Find Critical Points
Since slope fields map out differential equations, which are the derivatives of functions, we can use them to find the critical points—where the slope of a function is zero or undefined—of a particular solution.
To do this, we first need to identify regions where the line segments are horizontal and flat, indicating a slope of zero. These regions are potential candidates for critical points.
For example, in the slope field above for the differential equation , there are horizontal line segments along the line , meaning the slope is zero at those points. In the differential equation, when x and y are the same, subtracting them yields a difference of zero, so those points are candidates for critical points.
Critical points also occur when the slope is undefined, which is represented by a vertical line in a slope field. In our example, there are lines that almost look vertical, but none are perfectly straight up, so there are no undefined slopes in this case.
🏁 Solutions as Functions or Families of Functions
When solving differential equations, the introduction of the constant of integration, often denoted as "," is a key element that gives rise to a family of functions. The constant of integration represents an arbitrary constant value that gets added during the integration process. Why is this important? It's because it leads to not just one solution, but a whole group of possible solutions, which we call a family of functions. 👪
Finding the solution of a differential equation requires us to find the indefinite integral of the equation, which includes the constant of integration. This constant accounts for the fact that the derivative of a constant is always zero, and when integrating, we lose information about the specific value of the constant. Its inclusion acknowledges that there are numerous possibilities for the specific function that satisfies the differential equation. Essentially, the constant of integration allows for a wide range of solutions, each differing by the value of this constant.
The introduction of the arbitrary constant leads to a family of functions because, for each unique value of "+C," we obtain a distinct solution to the differential equation. The family encompasses all possible solutions, forming a spectrum of functions with varying characteristics. To pinpoint a particular solution from the family, we often need additional information or initial conditions. These conditions provide specific values that, when substituted into the general solution with the "+C," uniquely determine the constant and, consequently, a particular function within the family. Below is a graph containing the family of solution curves to a differential equation. Each curve on its own is a particular solution with a specific constant in place of “+C” and together the curves constitute the family of functions that are solutions to the differential equation.

Image courtesy of BYJU’s.
Each curve contains the same parent function, but each curve is slightly different due to “+C” being replaced by -1, -2, -3, and so on.
✏️ Slope Fields Practice
Try applying some of this information below!
🎯 Critical Points
Given the slope field below, find all possible critical points of the original function.

Image courtesy of PBworks.
Recall that critical points occur when the slope of a function is zero or undefined. Since a differential equation models the slope of a function, we simply need to find when there are horizontal line segments in the slope field where the slope would be zero, and when there are vertical line segments that show the slope at that point is undefined.
In the slope field above, we can see that along the line , there are horizontal line segments, indicating the slope is zero. Upon further examination, some of the lines come close to being vertical, but there are no vertical lines present in the slope field.
From this information, we can conclude that possible critical points lie along the line .
🏁 Family of Functions
Let’s solve the differential equation and graph its solutions to see how a family of functions is created.
This differential equation is the derivative of . If you didn’t know that, be sure to review your trig derivatives and inverse trig derivatives! As always when taking a definite integral, we have to include a “+C” to represent the constant of integration because this differential equation could be the derivative of , , or any value of C. So, our solution to this differential equation becomes .
Since C could be any real number, let’s graph our solution curve with different values of C to create a family of functions.

Image courtesy of Desmos.
As you can see, the solution to the differential curve could be any one of the above functions since we were not given an initial value that would allow us to find a particular solution.
✨ Closing
To sum it up, we've covered slope fields and how they play a role in understanding differential equations visually. We also explored how solving differential equations introduces the "+C" constant, leading to a family of functions. Each solution in the family is a bit different due to this constant. We emphasized the need for extra info or initial conditions to pin down a specific solution within the family. 📚

© 2024 Fiveable Inc. All rights reserved.