Browse By Unit
Fiveable SAT Math: Formulas to Memorize
11 min read•july 16, 2024
SAT Math: Essential Formulas To Memorize
Looking for a list of formulas to memorize for the SAT Math section? 👀
You're in the right place! We've compiled a list of formulas that are helpful to memorize when tackling the SAT Math section. Let's get into it and break down each formula, grab your notebook! 📒
**It is important to note that while the math reference sheet provided in the SAT is incredibly helpful, it doesn't include all the formulas you'll need to know. So, while the reference sheet provides a solid starting point, our goal is to dive deeper into the world of math formulas and thereby successfully prep you for that rigorous SAT math section. **
SAT Math: Linear Line Formulas
First, we're going to dive into some formulas for straight lines. Linear equations can exist in three main forms, and it's important to know when to use which to help you answer a question!
The Standard Form of Linear Equations
🏹 Standard form: Ax + By = C
-
- "A": coefficient of x
-
- "B": coefficient of y
-
- "x" and "y": variables
-
- "C": constant 🤔The standard form of a linear equation represents a line as a combination of x and y variables with coefficients (A and B) and a constant (C). It provides a general form for linear equations, but it is often rearranged to other forms for easier interpretation.
The Slope-Intercept Form of Linear Equations
🏹Slope-Intercept form: y= mx+b
-
- "m": slope of the line
-
- "y" and "x": variables
-
- "b": y-intercept of the line 🤔The slope-intercept form is a commonly used representation of a linear equation. It shows the relationship between the y-coordinate and the x-coordinate on the line. The slope (m) represents the line's steepness, and the y-intercept (b) is the point where the line intersects the y-axis.
The Point-Slope Form of Linear Equations
🏹Point-Slope form: y - y₁ = m(x - x₁)
-
- "x₁" and "y₁" : coordinates of a given point
-
- "m": slope
-
- "y" and "x": variables 🤔The point-slope form of a linear equation (y - y₁ = m(x - x₁)) is useful for determining the equation of a line when the slope (m) and a point (x₁, y₁) on the line are known.
The Slope of Linear Lines
Remember how slope equals rise/run? Or m=rise/run? Let's take a look at what this really means!
🏹Slope of a linear line: (y₂ - y₁) / (x₂ - x₁)
-
- "y₁" and "x₁": the coordinates of the first point on the line
-
- "y₂" and "x₂": the coordinates of the second point on the line- - It really doesn't matter which point comes first and which comes second, just make sure that x₁ and y₁ are the coordinates of the same point; and vice versa for x₂ and y₂. 🤔The slope formula calculates the slope of a line between two points (x₁, y₁) and (x₂, y₂) by finding the ratio of the vertical change (rise) to the horizontal change (run).
The Midpoint Formula
🏹Midpoint formula: ( (x₁ + x₂) / 2 , (y₁ + y₂) / 2)
-
- (x₁ + x₂) / 2 is used to find the x-coordinate while the (y₁ + y₂) / 2 is used to find the y-coordinate of the midpoint. The answer should be written in (x,y) form.
-
- "y₁" and "x₁": the coordinates of the first point on the line
-
- "y₂" and "x₂": the coordinates of the second point on the line 🤔The midpoint formula helps find the coordinates of the midpoint between two given points. By averaging the x-coordinates and the y-coordinates of the two points, we can determine the coordinates of the midpoint. This formula allows us to determine the center point or middle point on a line segment.
The Distance Formula
🏹Distance Formula: √((x₂ - x₁)² + (y₂ - y₁)²)
-
- "y₁" and "x₁": the coordinates of the first point on the line
-
- "y₂" and "x₂": the coordinates of the second point on the line 🤔The distance formula calculates the distance between two points on a coordinate plane. By using the coordinates of the two points, it determines the length of the line segment connecting them. This formula utilizes the Pythagorean theorem to find the square root of the sum of the squares of the differences between the x-coordinates and the y-coordinates.
SAT Math: Distance/Rate Formula
🏹 Distance = Speed × Time
-
- "Distance": the total distance traveled by the object
-
- "Speed": the rate at which the object is moving
-
- "Time": the duration of the travel 🤔The distance/rate formula is a fundamental formula used to calculate the distance traveled by an object based on its speed and the time it takes to travel. By using this formula, we can analyze and solve various problems related to distance, speed, and time. It is commonly applied in physics, everyday travel calculations, and other fields where measuring and understanding distances and rates of motion are important.
You may be wondering what the difference is between this distance formula and the one we included in the Linear Line formulas! Essentially, the linear line distance formula helps us find the distance between two points on a graph, while this distance formula is used when calculating the distance traveled by an object when it moves at a constant speed.
SAT Math: Quadratic Equations/Parabolas
Just like the linear line equations, there are several forms of quadratic equations. Let's dig in! 🤓
Standard Form of a Quadratic Equation
🏹Standard form: ax² + bx + c = 0
-
- "a", "b", and "c": constants- - "a" determines the shape of the parabolic curve. If "a" is positive, the parabola opens upward, and if "a" is negative, it opens downward.- - "b" and "c" affect the position and orientation of the parabola.
-
- "x": variable 🤔The standard/quadratic form of a quadratic equation represents a parabola as a quadratic expression equal to zero. The constants "a," "b," and "c" determine the shape, position, and orientation of the parabola. The coefficient "a" determines whether the parabola opens upward or downward, while "b" and "c" affect its position and orientation.
The Vertex Form of a Quadratic Equation
🏹 Vertex form: y = a(x - h)² + k
-
- "a": coefficient of the quadratic term
-
- "(h, k)": the coordinates of the vertex- - h: x-coordinate of the vertex- - k: y-coordinate of the vertex
-
- "x": variable 🤔The vertex form of a quadratic equation represents a parabola in terms of its vertex coordinates, (h, k), and the coefficient "a." The vertex (h, k) is the point where the parabola reaches its maximum or minimum value, depending on whether "a" is positive or negative.
The Factored Form of a Quadratic Equation
🏹 Factored form: y = a(x - r₁)(x - r₂)
-
- "a": coefficient of the quadratic term
-
- "r₁" and "r₂": the roots or solutions of the quadratic equation- - "r₁" and "r₂" represent the x-coordinates where the graph intersects the x-axis. In simple terms, the roots are the values of "x" that make the equation equal to zero. 🤔The factored form of a quadratic equation represents the equation as a product of linear factors (x - r₁)(x - r₂), where "r₁" and "r₂" are the roots or solutions of the equation. These roots are the x-coordinates where the graph of the quadratic equation intersects the x-axis, meaning they are the values of "x" that make the equation equal to zero. The factored form allows us to easily identify the roots and understand how the quadratic equation is factored.
Coordinates of the Vertex in a Parabola
🏹The x-Coordinate of the Vertex: x = -b / (2a)
🏹The y-Coordinate of the Vertex: y = f( -b / (2a))
-
- Here, "a" and "b" are the coefficients of the quadratic equation: ax²+by+c. The x-coordinate of the vertex represents the axis of symmetry of the parabola and is obtained by dividing "-b" by 2 times the coefficient "a".
-
- To find the y-coordinate of the vertex, plug in the x-coordinate obtained above into the equation as x. The resulting value will give you the y-coordinate of the vertex. 🤔The coordinates of the vertex provide information about the vertex of the parabolic curve represented by the quadratic equation:
-
- The x-coordinate of the vertex, given by x = -b / (2a), represents the axis of symmetry of the parabola. It is obtained by dividing the negative coefficient "b" by 2 times the coefficient "a."
-
- The y-coordinate of the vertex, denoted as y = f(-b / (2a)), is obtained by substituting the x-coordinate into the original equation. This provides the corresponding y-value of the vertex.
SAT Math: Circle Formulas
Arc Length Formula: L = 2πr (θ/360)
-
- "L": length
-
- "r": radius
-
- "θ": the measure of the central angle subtended by the arc
** The arc length formula calculates the length (L) of an arc on a circle based on the radius (r) and the measure of the central angle (θ) subtended by that arc. It relates the length of the arc to the circumference of the circle.**
Sector Area Formula: A = (θ/360)πr²
-
- "A": area
-
- "r": radius
-
- "θ": measure of the central angle subtended by the sector
** The sector area formula calculates the area (A) of a sector of a circle using the radius (r) and the measure of the central angle (θ) subtended by that sector. It relates the area of the sector to the total area of the circle.**
Center-Radius Equation: (x - h)² + (y - k)² = r²
-
- "(h, k)": coordinates of the center of the circle- - "h": x-coordinate- - "k": y-coordinate
-
- "r": the radius—the distance from the center to any point on the circle.
-
- "x" and "y": variables
** The center-radius equation represents the equation of a circle with its center at the point (h, k) and a radius (r). It shows the relationship between the coordinates (x, y) on the circle and the distance from the center to any point on the circle.**
SAT Math: Exponents/ Roots Formulas
Product of Powers: a^m * a^n = a^(m + n)
-
- "a": the base
-
- "m" and "n": the exponents or powers associated with that base
** The product of powers formula states that when you multiply two powers with the same base (a^m * a^n), you can add their exponents to obtain the result (a^(m + n)). It allows for the consolidation of like terms and simplifies the expression. **
Power of a Power: (a^m)^n = a^(m * n)
-
- "a": the base
-
- "m" and "n": the exponents or powers associated with that base
** The power of a power formula states that when you raise a power to another exponent ((a^m)^n), you can multiply the exponents to obtain the result (a^(m * n)). It demonstrates the relationship between nested exponents and simplifies the expression.**
Power of a Product: (a * b)^n = a^n * b^n
-
- "a" and "b": the base
-
- "n": the exponent associated with the base
** The power of a product formula states that when you raise a product of bases to an exponent ((a * b)^n), you can distribute the exponent to each base to obtain the result (a^n * b^n). It allows for the individual evaluation of powers on each base and simplifies the expression.**
Quotient of Powers: a^m / a^n = a^(m - n)
-
- "a": the base
-
- "m" and "n": the exponents or powers associated with that base
** The quotient of powers formula states that when you divide two powers with the same base (a^m / a^n), you can subtract the exponents to obtain the result (a^(m - n)). This formula allows you to simplify expressions involving the division of powers with the same base by subtracting their exponents.**
Negative Exponent: a^(-n) = 1 / a^n
-
- "a": the base
-
- "n": the exponent associated with the base
** The negative exponent formula states that when you have a negative exponent (a^(-n)), you can rewrite it as the reciprocal of the base raised to the positive exponent (1 / a^n). This formula allows you to convert a negative exponent into a positive exponent and express the result as a fraction. **
SAT Math: Exponential Function/ Compound Interest
General form of an exponential function: f(x) = a * b^x
-
- "f(x)": represents the output or value of the function at a given input
-
- "x": input
-
- "a" : the initial value or y-intercept of the function
-
- "b": the base of the exponential function- - If the base "b" is greater than 1, the function exhibits exponential growth as "x" increases.- - If the base "b" is between 0 and 1, the function shows exponential decay as "x" increases.- - If the base "b" is equal to 1, the function becomes a constant function with a horizontal line.
** The general form of an exponential function represents a mathematical relationship between an input (x) and its corresponding output or value (f(x)). **
Compound interest formula: A = P(1 + r/n)^(nt)
** Exponential functions find various applications, and one notable example is compound interest. In the context of compound interest, the general form of an exponential function is used to model the growth of an investment over time.**
-
- "A": total amount
-
- "P": the principal (initial) amount
-
- "r": the annual interest rate (expressed as a decimal),
-
- "n": the number of compounding periods per year
-
- "t": the number of year
Continuous compound interest formula: A = P * e^(rt)
** The continuous compound interest formula is used to calculate the total amount (A) accumulated through continuous compounding of interest. By applying the continuous compound interest formula, you can determine the future value of an investment that earns continuous compounding interest over time. Continuous compounding assumes that the interest is constantly reinvested and compounded without any specific intervals or discrete periods.**
-
- "A": total amount
-
- "P": the principal (initial) amount
-
- "e": Euler's number (approximately 2.71828)
-
- "r": the annual interest rate (expressed as a decimal)
-
- "t": the number of year
SAT Math: Trigonometry Functions
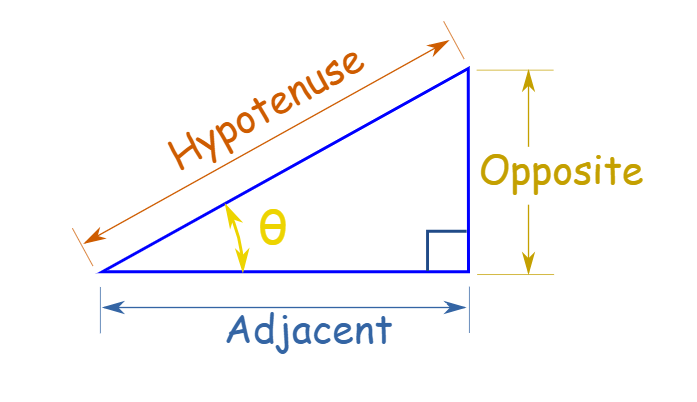
Image Courtesy of MathsisFun.
Sine (sin): The sine of an angle is the length of the side opposite to the angle divided by the length of the hypotenuse.
-
- sin(θ) = opposite/hypotenuse
Cosine (cos): The cosine of an angle is the length of the side adjacent to the angle divided by the length of the hypotenuse.
-
- cos(θ) = adjacent/hypotenuse
Tangent (tan): The tangent of an angle is the length of the side opposite the angle divided by the length of the side adjacent to the angle.
-
- tan(θ) = opposite/adjacent
Cosecant (csc): The cosecant of an angle is the reciprocal of the sine of the angle.
-
- csc(θ) = 1/sin(θ)
Secant (sec): The secant of an angle is the reciprocal of the cosine of the angle
-
- sec(θ) = 1/cos(θ)
Cotangent (cot): The cotangent of an angle is the reciprocal of the tangent of the angle.
-
- cot(θ) = 1/tan(θ)
And there you have it! We've explored a variety of important math formulas that will not only help you excel in the SAT but also expand your mathematical toolkit. So go forth, my math-savvy friend, and let the formulas guide you toward success! Good luck, and may your SAT experience be filled with joy and achievement.
<< Hide Menu
Fiveable SAT Math: Formulas to Memorize
11 min read•july 16, 2024
SAT Math: Essential Formulas To Memorize
Looking for a list of formulas to memorize for the SAT Math section? 👀
You're in the right place! We've compiled a list of formulas that are helpful to memorize when tackling the SAT Math section. Let's get into it and break down each formula, grab your notebook! 📒
**It is important to note that while the math reference sheet provided in the SAT is incredibly helpful, it doesn't include all the formulas you'll need to know. So, while the reference sheet provides a solid starting point, our goal is to dive deeper into the world of math formulas and thereby successfully prep you for that rigorous SAT math section. **
SAT Math: Linear Line Formulas
First, we're going to dive into some formulas for straight lines. Linear equations can exist in three main forms, and it's important to know when to use which to help you answer a question!
The Standard Form of Linear Equations
🏹 Standard form: Ax + By = C
-
- "A": coefficient of x
-
- "B": coefficient of y
-
- "x" and "y": variables
-
- "C": constant 🤔The standard form of a linear equation represents a line as a combination of x and y variables with coefficients (A and B) and a constant (C). It provides a general form for linear equations, but it is often rearranged to other forms for easier interpretation.
The Slope-Intercept Form of Linear Equations
🏹Slope-Intercept form: y= mx+b
-
- "m": slope of the line
-
- "y" and "x": variables
-
- "b": y-intercept of the line 🤔The slope-intercept form is a commonly used representation of a linear equation. It shows the relationship between the y-coordinate and the x-coordinate on the line. The slope (m) represents the line's steepness, and the y-intercept (b) is the point where the line intersects the y-axis.
The Point-Slope Form of Linear Equations
🏹Point-Slope form: y - y₁ = m(x - x₁)
-
- "x₁" and "y₁" : coordinates of a given point
-
- "m": slope
-
- "y" and "x": variables 🤔The point-slope form of a linear equation (y - y₁ = m(x - x₁)) is useful for determining the equation of a line when the slope (m) and a point (x₁, y₁) on the line are known.
The Slope of Linear Lines
Remember how slope equals rise/run? Or m=rise/run? Let's take a look at what this really means!
🏹Slope of a linear line: (y₂ - y₁) / (x₂ - x₁)
-
- "y₁" and "x₁": the coordinates of the first point on the line
-
- "y₂" and "x₂": the coordinates of the second point on the line- - It really doesn't matter which point comes first and which comes second, just make sure that x₁ and y₁ are the coordinates of the same point; and vice versa for x₂ and y₂. 🤔The slope formula calculates the slope of a line between two points (x₁, y₁) and (x₂, y₂) by finding the ratio of the vertical change (rise) to the horizontal change (run).
The Midpoint Formula
🏹Midpoint formula: ( (x₁ + x₂) / 2 , (y₁ + y₂) / 2)
-
- (x₁ + x₂) / 2 is used to find the x-coordinate while the (y₁ + y₂) / 2 is used to find the y-coordinate of the midpoint. The answer should be written in (x,y) form.
-
- "y₁" and "x₁": the coordinates of the first point on the line
-
- "y₂" and "x₂": the coordinates of the second point on the line 🤔The midpoint formula helps find the coordinates of the midpoint between two given points. By averaging the x-coordinates and the y-coordinates of the two points, we can determine the coordinates of the midpoint. This formula allows us to determine the center point or middle point on a line segment.
The Distance Formula
🏹Distance Formula: √((x₂ - x₁)² + (y₂ - y₁)²)
-
- "y₁" and "x₁": the coordinates of the first point on the line
-
- "y₂" and "x₂": the coordinates of the second point on the line 🤔The distance formula calculates the distance between two points on a coordinate plane. By using the coordinates of the two points, it determines the length of the line segment connecting them. This formula utilizes the Pythagorean theorem to find the square root of the sum of the squares of the differences between the x-coordinates and the y-coordinates.
SAT Math: Distance/Rate Formula
🏹 Distance = Speed × Time
-
- "Distance": the total distance traveled by the object
-
- "Speed": the rate at which the object is moving
-
- "Time": the duration of the travel 🤔The distance/rate formula is a fundamental formula used to calculate the distance traveled by an object based on its speed and the time it takes to travel. By using this formula, we can analyze and solve various problems related to distance, speed, and time. It is commonly applied in physics, everyday travel calculations, and other fields where measuring and understanding distances and rates of motion are important.
You may be wondering what the difference is between this distance formula and the one we included in the Linear Line formulas! Essentially, the linear line distance formula helps us find the distance between two points on a graph, while this distance formula is used when calculating the distance traveled by an object when it moves at a constant speed.
SAT Math: Quadratic Equations/Parabolas
Just like the linear line equations, there are several forms of quadratic equations. Let's dig in! 🤓
Standard Form of a Quadratic Equation
🏹Standard form: ax² + bx + c = 0
-
- "a", "b", and "c": constants- - "a" determines the shape of the parabolic curve. If "a" is positive, the parabola opens upward, and if "a" is negative, it opens downward.- - "b" and "c" affect the position and orientation of the parabola.
-
- "x": variable 🤔The standard/quadratic form of a quadratic equation represents a parabola as a quadratic expression equal to zero. The constants "a," "b," and "c" determine the shape, position, and orientation of the parabola. The coefficient "a" determines whether the parabola opens upward or downward, while "b" and "c" affect its position and orientation.
The Vertex Form of a Quadratic Equation
🏹 Vertex form: y = a(x - h)² + k
-
- "a": coefficient of the quadratic term
-
- "(h, k)": the coordinates of the vertex- - h: x-coordinate of the vertex- - k: y-coordinate of the vertex
-
- "x": variable 🤔The vertex form of a quadratic equation represents a parabola in terms of its vertex coordinates, (h, k), and the coefficient "a." The vertex (h, k) is the point where the parabola reaches its maximum or minimum value, depending on whether "a" is positive or negative.
The Factored Form of a Quadratic Equation
🏹 Factored form: y = a(x - r₁)(x - r₂)
-
- "a": coefficient of the quadratic term
-
- "r₁" and "r₂": the roots or solutions of the quadratic equation- - "r₁" and "r₂" represent the x-coordinates where the graph intersects the x-axis. In simple terms, the roots are the values of "x" that make the equation equal to zero. 🤔The factored form of a quadratic equation represents the equation as a product of linear factors (x - r₁)(x - r₂), where "r₁" and "r₂" are the roots or solutions of the equation. These roots are the x-coordinates where the graph of the quadratic equation intersects the x-axis, meaning they are the values of "x" that make the equation equal to zero. The factored form allows us to easily identify the roots and understand how the quadratic equation is factored.
Coordinates of the Vertex in a Parabola
🏹The x-Coordinate of the Vertex: x = -b / (2a)
🏹The y-Coordinate of the Vertex: y = f( -b / (2a))
-
- Here, "a" and "b" are the coefficients of the quadratic equation: ax²+by+c. The x-coordinate of the vertex represents the axis of symmetry of the parabola and is obtained by dividing "-b" by 2 times the coefficient "a".
-
- To find the y-coordinate of the vertex, plug in the x-coordinate obtained above into the equation as x. The resulting value will give you the y-coordinate of the vertex. 🤔The coordinates of the vertex provide information about the vertex of the parabolic curve represented by the quadratic equation:
-
- The x-coordinate of the vertex, given by x = -b / (2a), represents the axis of symmetry of the parabola. It is obtained by dividing the negative coefficient "b" by 2 times the coefficient "a."
-
- The y-coordinate of the vertex, denoted as y = f(-b / (2a)), is obtained by substituting the x-coordinate into the original equation. This provides the corresponding y-value of the vertex.
SAT Math: Circle Formulas
Arc Length Formula: L = 2πr (θ/360)
-
- "L": length
-
- "r": radius
-
- "θ": the measure of the central angle subtended by the arc
** The arc length formula calculates the length (L) of an arc on a circle based on the radius (r) and the measure of the central angle (θ) subtended by that arc. It relates the length of the arc to the circumference of the circle.**
Sector Area Formula: A = (θ/360)πr²
-
- "A": area
-
- "r": radius
-
- "θ": measure of the central angle subtended by the sector
** The sector area formula calculates the area (A) of a sector of a circle using the radius (r) and the measure of the central angle (θ) subtended by that sector. It relates the area of the sector to the total area of the circle.**
Center-Radius Equation: (x - h)² + (y - k)² = r²
-
- "(h, k)": coordinates of the center of the circle- - "h": x-coordinate- - "k": y-coordinate
-
- "r": the radius—the distance from the center to any point on the circle.
-
- "x" and "y": variables
** The center-radius equation represents the equation of a circle with its center at the point (h, k) and a radius (r). It shows the relationship between the coordinates (x, y) on the circle and the distance from the center to any point on the circle.**
SAT Math: Exponents/ Roots Formulas
Product of Powers: a^m * a^n = a^(m + n)
-
- "a": the base
-
- "m" and "n": the exponents or powers associated with that base
** The product of powers formula states that when you multiply two powers with the same base (a^m * a^n), you can add their exponents to obtain the result (a^(m + n)). It allows for the consolidation of like terms and simplifies the expression. **
Power of a Power: (a^m)^n = a^(m * n)
-
- "a": the base
-
- "m" and "n": the exponents or powers associated with that base
** The power of a power formula states that when you raise a power to another exponent ((a^m)^n), you can multiply the exponents to obtain the result (a^(m * n)). It demonstrates the relationship between nested exponents and simplifies the expression.**
Power of a Product: (a * b)^n = a^n * b^n
-
- "a" and "b": the base
-
- "n": the exponent associated with the base
** The power of a product formula states that when you raise a product of bases to an exponent ((a * b)^n), you can distribute the exponent to each base to obtain the result (a^n * b^n). It allows for the individual evaluation of powers on each base and simplifies the expression.**
Quotient of Powers: a^m / a^n = a^(m - n)
-
- "a": the base
-
- "m" and "n": the exponents or powers associated with that base
** The quotient of powers formula states that when you divide two powers with the same base (a^m / a^n), you can subtract the exponents to obtain the result (a^(m - n)). This formula allows you to simplify expressions involving the division of powers with the same base by subtracting their exponents.**
Negative Exponent: a^(-n) = 1 / a^n
-
- "a": the base
-
- "n": the exponent associated with the base
** The negative exponent formula states that when you have a negative exponent (a^(-n)), you can rewrite it as the reciprocal of the base raised to the positive exponent (1 / a^n). This formula allows you to convert a negative exponent into a positive exponent and express the result as a fraction. **
SAT Math: Exponential Function/ Compound Interest
General form of an exponential function: f(x) = a * b^x
-
- "f(x)": represents the output or value of the function at a given input
-
- "x": input
-
- "a" : the initial value or y-intercept of the function
-
- "b": the base of the exponential function- - If the base "b" is greater than 1, the function exhibits exponential growth as "x" increases.- - If the base "b" is between 0 and 1, the function shows exponential decay as "x" increases.- - If the base "b" is equal to 1, the function becomes a constant function with a horizontal line.
** The general form of an exponential function represents a mathematical relationship between an input (x) and its corresponding output or value (f(x)). **
Compound interest formula: A = P(1 + r/n)^(nt)
** Exponential functions find various applications, and one notable example is compound interest. In the context of compound interest, the general form of an exponential function is used to model the growth of an investment over time.**
-
- "A": total amount
-
- "P": the principal (initial) amount
-
- "r": the annual interest rate (expressed as a decimal),
-
- "n": the number of compounding periods per year
-
- "t": the number of year
Continuous compound interest formula: A = P * e^(rt)
** The continuous compound interest formula is used to calculate the total amount (A) accumulated through continuous compounding of interest. By applying the continuous compound interest formula, you can determine the future value of an investment that earns continuous compounding interest over time. Continuous compounding assumes that the interest is constantly reinvested and compounded without any specific intervals or discrete periods.**
-
- "A": total amount
-
- "P": the principal (initial) amount
-
- "e": Euler's number (approximately 2.71828)
-
- "r": the annual interest rate (expressed as a decimal)
-
- "t": the number of year
SAT Math: Trigonometry Functions

Image Courtesy of MathsisFun.
Sine (sin): The sine of an angle is the length of the side opposite to the angle divided by the length of the hypotenuse.
-
- sin(θ) = opposite/hypotenuse
Cosine (cos): The cosine of an angle is the length of the side adjacent to the angle divided by the length of the hypotenuse.
-
- cos(θ) = adjacent/hypotenuse
Tangent (tan): The tangent of an angle is the length of the side opposite the angle divided by the length of the side adjacent to the angle.
-
- tan(θ) = opposite/adjacent
Cosecant (csc): The cosecant of an angle is the reciprocal of the sine of the angle.
-
- csc(θ) = 1/sin(θ)
Secant (sec): The secant of an angle is the reciprocal of the cosine of the angle
-
- sec(θ) = 1/cos(θ)
Cotangent (cot): The cotangent of an angle is the reciprocal of the tangent of the angle.
-
- cot(θ) = 1/tan(θ)
And there you have it! We've explored a variety of important math formulas that will not only help you excel in the SAT but also expand your mathematical toolkit. So go forth, my math-savvy friend, and let the formulas guide you toward success! Good luck, and may your SAT experience be filled with joy and achievement.

© 2024 Fiveable Inc. All rights reserved.