Browse By Unit
3.3 Sine and Cosine Function Values
6 min read•june 18, 2024
Kashvi Panjolia
Kashvi Panjolia
Understanding the Unit Circle
The unit circle is a special kind of circle that has a radius of 1 and is centered at the origin (0,0) on a coordinate plane. It might not seem like much at first glance, but this simple circle is the key to understanding a whole host of important concepts in pre-calculus, from trigonometry to polar coordinates. 🔑
To begin, we need to understand the concept of an angle in standard position. An angle in standard position is an angle measured starting from the positive x-axis and going counterclockwise. ⏰
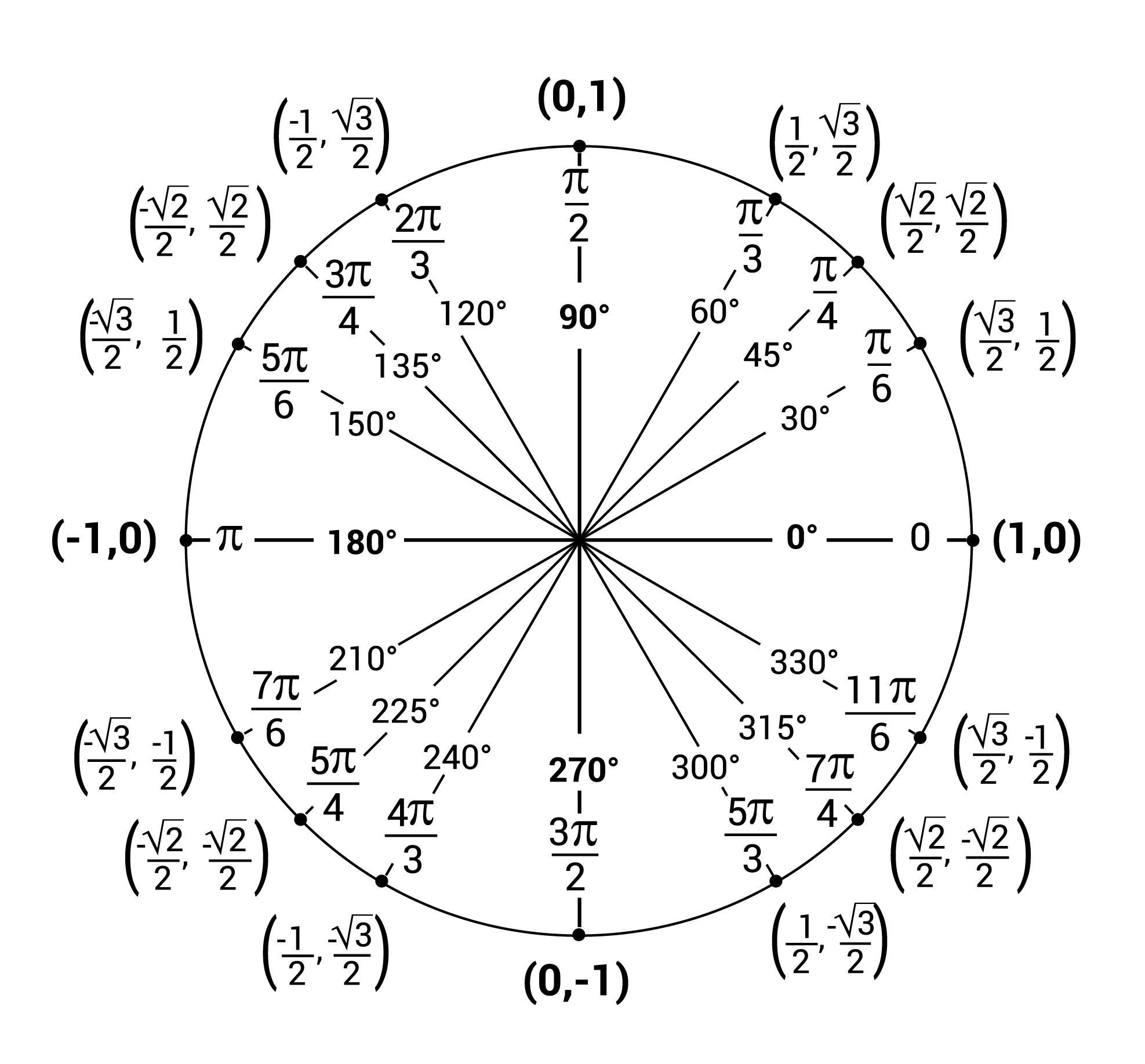
Image courtesy of Mometrix Test Preparation.
Above is an image of the unit circle. It may look very complicated at first glance, but you'll get very familiar with the unit circle in this unit, and you will realize that it's not that complicated after all. This circle has a radius of 1 unit on the coordinate plane -- that's why the points at which it intersects the x- and y- axes are variations of the point (1,0). We look at the unit circle starting not at the top, but at the right, on the positive x-axis. Here, we can see that the angle that is written on the circle is 0 degrees (or 0 radians).
Finding Angles on the Unit Circle
To make a full rotation of the unit circle, we start at 0 degrees and look counterclockwise until we get back to the positive x-axis. As you go around the circle, there are values written in radians and their corresponding values in degrees on the inside of the circle. These values are the measures of the angle the ray they are on makes with the positive x-axis, measured in standard position. 📐
For example, 𝞹/6 in radians represents that the angle made by the ray it lies on and the positive x-axis is 𝞹/6 radians, or 30 degrees. 300 degrees, or 5𝞹/3 radians, represents that the angle the ray it lies on makes with the positive x-axis is 300 degrees when measured counterclockwise from the positive x-axis. We can see that this is a reflex angle (an angle that measures greater than 180 degrees but less than 360 degrees) and not the most efficient way to measure this angle. An angle of 5𝞹/3 radians counterclockwise is the same as an angle of -𝞹/3 radians. This angle is now negative because we are measuring it clockwise from the positive x-axis, so we are going down to 5𝞹/3, rather than all the way around the circle. However, both 5𝞹/3 and -𝞹/3 still identify the same angle on the unit circle.
Another important feature of the unit circle is that one full revolution around it is equal to 2𝞹 radians, or 360 degrees. You can revolve around the unit circle as many times as you want, increasing the value of your angle by 2𝞹 radians each time. For example, if you were to make 3 and a half revolutions of the unit circle, you would multiply 2𝞹 by 3, yielding 6𝞹, then add a 𝞹 to that for the half-circle. You will have covered 7𝞹 radians in 3 and a half revolutions.
Trigonometry with the Unit Circle
When we take an angle in standard position and draw a line (called the "terminal ray") that goes out from the origin at that angle, the point where this line intersects the unit circle is called Point P. The coordinates of Point P are given by (cosθ, sinθ), where θ is the measure of the angle in radians or degrees. These coordinates are based on the trigonometric functions sine and cosine, which are used to describe the relationships between the angles and sides of a right triangle. The sine of an angle is the y-coordinate of the point on the unit circle, and the cosine is the x-coordinate. The tangent of an angle is the ratio of the y-coordinate to the x-coordinate, or tan(θ) = sin(θ) / cos(θ).
To find the sine, cosine, or tangent values at a specific angle, we can simply use the coordinates of Point P. For example, if we want to find the sine of 30 degrees, we can draw the angle in standard position on the unit circle, and find the point of intersection, P. The y-coordinate of P is the sine value at 30 degrees, which is 0.5. Similarly, the x-coordinate of P is the cosine value at 30 degrees, which is √3/2. And the tangent value at 30 degrees is 0.5/ √3/2 = √3/3.
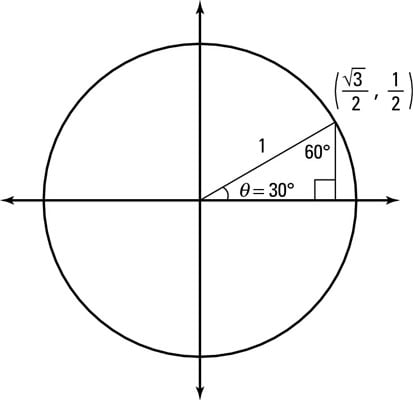
Image courtesy of Dummies.
It's important to note that these values remain constant regardless of the size of the right triangle. These values are called the "unit circle's ratios" and they can be used to find the values of sine, cosine and tangent for any angle. ◢
** You can use the acronym SOHCAHTOA to remember how to find the sine, cosine, and tangent of an angle when given a right triangle. SOH = Sine is the value of the side Opposite the angle over the value of the Hypotenuse. CAH = Cosine is the value of the side Adjacent to the angle over the Hypotenuse. TOA = Tangent is the side Opposite the angle over the Hypotenuse.**
As you can see in the image above, the unit circle is created using the side lengths of the right triangles created by various angles. The hypotenuse of a triangle on the unit circle will always be 1 because that is the radius of the circle. The horizontal side of the triangle is used to find the cosine of the angle θ, so it is the x-coordinate of point P. The vertical side of the triangle is used to find the sine of the angle θ, so it is the y-coordinate of point P.
Finding the Angle Given the Trig Value
Using the unit circle, we can also find the angle given the sine or cosine value. If we are given that the cosine of the angle we are looking for is 1/2, we can find where 1/2 is an x-coordinate on the unit circle, since we know that cosine values are the x-coordinates. Starting at the 0-degree mark, or the positive x-axis, we can work our way around the unit circle counterclockwise until we find an x-coordinate of 1/2. Very quickly, we would see that 1/2 is an x-coordinate when the angle is 𝞹/3 radians, or 60 degrees.
If we are given that the sine of the angle is -√3/2 and are asked to find the angle, we would follow a similar process as before. Starting at the positive x-axis and moving counterclockwise, we now look at the y-values on the unit circle to find -√3/2. We find what we are looking for in the 3rd quadrant (bottom left) of the coordinate plane, at an angle of 4𝞹/3 radians.
Determining the Sign of Trig Values
An important concept to learn is identifying when these trigonometric functions are positive or negative. ±
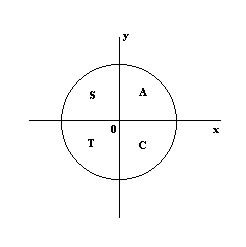
Image courtesy of RevisionMaths.
The acronym "All Students Take Calculus" is useful for remembering when each trigonometric function is positive.
All -- All the trig values (sine, cosine, and tangent) are positive in the first quadrant.
Students -- The Sine values are positive in the second quadrant, and all the other values are negative.
Take -- The Tangent values are positive in the third quadrant, and all the other values are negative.
Calculus -- The Cosine values are positive in the fourth quadrant, and all the other values are negative.
Take another look at the unit circle, and see how this acronym holds true.
<< Hide Menu
3.3 Sine and Cosine Function Values
6 min read•june 18, 2024
Kashvi Panjolia
Kashvi Panjolia
Understanding the Unit Circle
The unit circle is a special kind of circle that has a radius of 1 and is centered at the origin (0,0) on a coordinate plane. It might not seem like much at first glance, but this simple circle is the key to understanding a whole host of important concepts in pre-calculus, from trigonometry to polar coordinates. 🔑
To begin, we need to understand the concept of an angle in standard position. An angle in standard position is an angle measured starting from the positive x-axis and going counterclockwise. ⏰

Image courtesy of Mometrix Test Preparation.
Above is an image of the unit circle. It may look very complicated at first glance, but you'll get very familiar with the unit circle in this unit, and you will realize that it's not that complicated after all. This circle has a radius of 1 unit on the coordinate plane -- that's why the points at which it intersects the x- and y- axes are variations of the point (1,0). We look at the unit circle starting not at the top, but at the right, on the positive x-axis. Here, we can see that the angle that is written on the circle is 0 degrees (or 0 radians).
Finding Angles on the Unit Circle
To make a full rotation of the unit circle, we start at 0 degrees and look counterclockwise until we get back to the positive x-axis. As you go around the circle, there are values written in radians and their corresponding values in degrees on the inside of the circle. These values are the measures of the angle the ray they are on makes with the positive x-axis, measured in standard position. 📐
For example, 𝞹/6 in radians represents that the angle made by the ray it lies on and the positive x-axis is 𝞹/6 radians, or 30 degrees. 300 degrees, or 5𝞹/3 radians, represents that the angle the ray it lies on makes with the positive x-axis is 300 degrees when measured counterclockwise from the positive x-axis. We can see that this is a reflex angle (an angle that measures greater than 180 degrees but less than 360 degrees) and not the most efficient way to measure this angle. An angle of 5𝞹/3 radians counterclockwise is the same as an angle of -𝞹/3 radians. This angle is now negative because we are measuring it clockwise from the positive x-axis, so we are going down to 5𝞹/3, rather than all the way around the circle. However, both 5𝞹/3 and -𝞹/3 still identify the same angle on the unit circle.
Another important feature of the unit circle is that one full revolution around it is equal to 2𝞹 radians, or 360 degrees. You can revolve around the unit circle as many times as you want, increasing the value of your angle by 2𝞹 radians each time. For example, if you were to make 3 and a half revolutions of the unit circle, you would multiply 2𝞹 by 3, yielding 6𝞹, then add a 𝞹 to that for the half-circle. You will have covered 7𝞹 radians in 3 and a half revolutions.
Trigonometry with the Unit Circle
When we take an angle in standard position and draw a line (called the "terminal ray") that goes out from the origin at that angle, the point where this line intersects the unit circle is called Point P. The coordinates of Point P are given by (cosθ, sinθ), where θ is the measure of the angle in radians or degrees. These coordinates are based on the trigonometric functions sine and cosine, which are used to describe the relationships between the angles and sides of a right triangle. The sine of an angle is the y-coordinate of the point on the unit circle, and the cosine is the x-coordinate. The tangent of an angle is the ratio of the y-coordinate to the x-coordinate, or tan(θ) = sin(θ) / cos(θ).
To find the sine, cosine, or tangent values at a specific angle, we can simply use the coordinates of Point P. For example, if we want to find the sine of 30 degrees, we can draw the angle in standard position on the unit circle, and find the point of intersection, P. The y-coordinate of P is the sine value at 30 degrees, which is 0.5. Similarly, the x-coordinate of P is the cosine value at 30 degrees, which is √3/2. And the tangent value at 30 degrees is 0.5/ √3/2 = √3/3.

Image courtesy of Dummies.
It's important to note that these values remain constant regardless of the size of the right triangle. These values are called the "unit circle's ratios" and they can be used to find the values of sine, cosine and tangent for any angle. ◢
** You can use the acronym SOHCAHTOA to remember how to find the sine, cosine, and tangent of an angle when given a right triangle. SOH = Sine is the value of the side Opposite the angle over the value of the Hypotenuse. CAH = Cosine is the value of the side Adjacent to the angle over the Hypotenuse. TOA = Tangent is the side Opposite the angle over the Hypotenuse.**
As you can see in the image above, the unit circle is created using the side lengths of the right triangles created by various angles. The hypotenuse of a triangle on the unit circle will always be 1 because that is the radius of the circle. The horizontal side of the triangle is used to find the cosine of the angle θ, so it is the x-coordinate of point P. The vertical side of the triangle is used to find the sine of the angle θ, so it is the y-coordinate of point P.
Finding the Angle Given the Trig Value
Using the unit circle, we can also find the angle given the sine or cosine value. If we are given that the cosine of the angle we are looking for is 1/2, we can find where 1/2 is an x-coordinate on the unit circle, since we know that cosine values are the x-coordinates. Starting at the 0-degree mark, or the positive x-axis, we can work our way around the unit circle counterclockwise until we find an x-coordinate of 1/2. Very quickly, we would see that 1/2 is an x-coordinate when the angle is 𝞹/3 radians, or 60 degrees.
If we are given that the sine of the angle is -√3/2 and are asked to find the angle, we would follow a similar process as before. Starting at the positive x-axis and moving counterclockwise, we now look at the y-values on the unit circle to find -√3/2. We find what we are looking for in the 3rd quadrant (bottom left) of the coordinate plane, at an angle of 4𝞹/3 radians.
Determining the Sign of Trig Values
An important concept to learn is identifying when these trigonometric functions are positive or negative. ±

Image courtesy of RevisionMaths.
The acronym "All Students Take Calculus" is useful for remembering when each trigonometric function is positive.
All -- All the trig values (sine, cosine, and tangent) are positive in the first quadrant.
Students -- The Sine values are positive in the second quadrant, and all the other values are negative.
Take -- The Tangent values are positive in the third quadrant, and all the other values are negative.
Calculus -- The Cosine values are positive in the fourth quadrant, and all the other values are negative.
Take another look at the unit circle, and see how this acronym holds true.

© 2024 Fiveable Inc. All rights reserved.