Browse By Unit
AP Stats Mixed Units Practice FRQ #3 & Feedback
6 min read•july 11, 2024
Jerry Kosoff
Jerry Kosoff
Practicing with FRQs is a great way to prep for the AP exam! Review student responses for a FRQ combining multiple units and corresponding feedback from Fiveable teacher Jerry Kosoff.
The Mixed Units FRQ Prompt
A group of randomly-selected students at a large high school were given a survey about their transportation to school. One of the questions asked students for their primary mode of transportation; another asked students for the typical number of minutes it takes them to travel to school in the morning. Two groups of students are singled out for further comparison: those that said they walk to school as their primary mode of transportation, and those that said they drive to school as their primary mode of transportation. The two histograms below show the distribution of travel times, in minutes, for the two groups - though the group labels are not present. There are a similar number of students in each group.
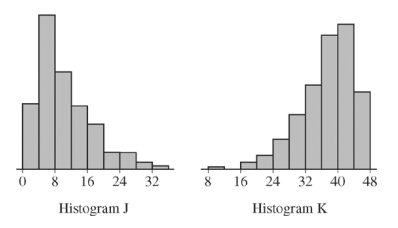
- Summary statistics for the two groups revealed that for the students who walk to school, the mean time it typically takes to get to school is lower than the median time it typically takes to get to school. Based on this information, which of the histograms shown above represents the group of students who walk to school? Justify your response.
- If the data from the two groups were combined into a single histogram, describe the shape of the resulting histogram.
- The distribution of travel times for the 500 staff members at the school shows a moderate right skew, with a mean of 25 minutes and a standard deviation of 18 minutes. For random samples of 36 staff members, describe the sampling distribution of sample mean travel times.
- A student wishes to determine if the mean travel time for teachers is different from the mean travel time for students at the school. To investigate, the student surveys 50 randomly-selected students and 40 randomly-selected teachers. State the name of the appropriate statistical test the student should conduct, and write appropriate hypotheses for the test you name. Be sure to define any parameters you use.
FRQ Writing Samples and Teacher Feedback
Student Response 1
-
Histogram K represents the group of students who walk to school because the mean is pulled towards outliers. Since Histogram K is skewed left, the mean would be pulled towards the skewness while the median would stay put as it is resistant to outliers. Histogram J however, shows the mean being greater than the median as it is being pulled to the right.
-
The resulting histogram of the combination of the two groups of students who walk to school and time it takes for school would be normally distributed.
-

-
Name of test: 2 sample t-test. We want to test a claim about the difference between mu1 (the true mean travel time it takes to travel to school for 50 students) and mu2 (the true mean travel time it takes to travel to school for 50 students.
Teacher Feedback
Your answer for part 1 is very good - you pick the correct histogram and give a clear reason for doing so (and clearly explain why it WOULDN’T be the other histogram). Full credit. For part 2, you say “normal,” which implies a unimodal graph with a peak in the center of the distribution. That won’t be the case here: if you look carefully at the x-axis for each distribution the peaks will end up separated (one around 8 minutes and the other around 40 minutes), whereas the center of the combined number line (around 24 minutes) has very few observations. Thus, bimodal is the description we’d be looking for here.
For part 3, your work is good - you’ve checked conditions, cited the use of the CLT, and correctly calculated the parameters of the sampling distribution. One thing - you never mention the shape (just saying “normality” as a condition isn’t equivalent to saying “the sampling distribution would be approximately normal.”). Therefore, you’d get partial instead of full credit. Advice - after citing the CLT, state that this means the sampling distribution is approximately normal.
Finally, in part 4, you’d also receive partial credit, if any. A 2-sample t-test is the correct test. However, you do not write fully correct statements for Ho and Ha. Your Ho would be that the mean travel times for the two groups are the same; Ha would be that the mean travel times for the groups are different. You only mention the ‘difference’ and do not cite it as the alternative hypothesis. Additionally, you define mu1 and mu2 as representing 50 students - but they would represent ALL students and ALL teachers at the school (since “mu” is a population parameter). I’m working on the assumption that you meant for one of your parameters to mention teachers. Be careful when typing/writing responses!
Student Response 2
- If the mean time is lower than the median time, that means the distribution is skewed to the left and would be appropriate for the distribution of histogram K. this is because when the distribution is skewed, the mean is pulled to the tail of the distribution and is either larger than or smaller than the median. In this case, for the mean to be smaller, the tail should be to the left.
- The distribution of both histograms J and k combined will have a bimodal shape and the peaks would be around 0-8 and 40-48.
- For a random sample of 36 staff members, the sampling distribution will follow a approximately normal distribution and have a mean of 25 minutes and a standard deviation of 18/ (square. root of 36) = 3 minutes. Because the sample size of 36 is larger than 30, it meets the CLT condition and can be assumed as approximately normal.
- The test would be two sample t test for the difference of means1. Ho : MUs - MUt =01. Ha : MUs - MUt does not equal 0.1. MUs represents the true mean travel time for students and MUt represents the true mean travel time for teachers/ staff
Teacher Feedback
All of your answers are correct and give appropriate reasoning. A tip for part (a): when asked to make a choice in AP stats, you should explain not only why your choice is correct, but why the other option is incorrect. You could add “since histogram J is right skewed, it does not match the description” or something similar. All of your other answers are thorough, in context, and show strong understanding. Well done!
Student Response 3
- If the mean is less than the median that means the data is skewed to the left, because the mean is affected by smaller values bringing it down. Histogram K appears skewed to the left, so it must represent the group of students who walk to school.
- The distribution can be described as bimodal.
- The shape of the histogram is approximately normal, because 36 is greater than 30, which satisfies the central limit theorem. The center is the mean, 25 minutes. The spread is the standard error, 18/sqrt36, or 3.
- Two sample means t test1. H0: mu1 = mu21. HA: mu1 is not equal to mu21. where mu1 is the mean travel time for students and mu2 is the mean travel time for teachers. Conditions:
- The students and teachers were randomly selected.
- 50 and 40 are both greater than 30, which satisfies the Central Limit Theorem, indicating that the distributions are approx. normal.
- There are at least 501 students and 401 teachers, so the 10% condition for independence is satisfied.
Teacher Feedback
Nice job! One small thing: for #2, you’re correct in calling the shape “bimodal,” but the rubric for a similar question only gave full credit for identifying where the clusters/peaks were centered. So you’d get partial credit. Full credit: “bimodal, with peaks at around 8 minutes and 40 minutes”
<< Hide Menu
AP Stats Mixed Units Practice FRQ #3 & Feedback
6 min read•july 11, 2024
Jerry Kosoff
Jerry Kosoff
Practicing with FRQs is a great way to prep for the AP exam! Review student responses for a FRQ combining multiple units and corresponding feedback from Fiveable teacher Jerry Kosoff.
The Mixed Units FRQ Prompt
A group of randomly-selected students at a large high school were given a survey about their transportation to school. One of the questions asked students for their primary mode of transportation; another asked students for the typical number of minutes it takes them to travel to school in the morning. Two groups of students are singled out for further comparison: those that said they walk to school as their primary mode of transportation, and those that said they drive to school as their primary mode of transportation. The two histograms below show the distribution of travel times, in minutes, for the two groups - though the group labels are not present. There are a similar number of students in each group.

- Summary statistics for the two groups revealed that for the students who walk to school, the mean time it typically takes to get to school is lower than the median time it typically takes to get to school. Based on this information, which of the histograms shown above represents the group of students who walk to school? Justify your response.
- If the data from the two groups were combined into a single histogram, describe the shape of the resulting histogram.
- The distribution of travel times for the 500 staff members at the school shows a moderate right skew, with a mean of 25 minutes and a standard deviation of 18 minutes. For random samples of 36 staff members, describe the sampling distribution of sample mean travel times.
- A student wishes to determine if the mean travel time for teachers is different from the mean travel time for students at the school. To investigate, the student surveys 50 randomly-selected students and 40 randomly-selected teachers. State the name of the appropriate statistical test the student should conduct, and write appropriate hypotheses for the test you name. Be sure to define any parameters you use.
FRQ Writing Samples and Teacher Feedback
Student Response 1
-
Histogram K represents the group of students who walk to school because the mean is pulled towards outliers. Since Histogram K is skewed left, the mean would be pulled towards the skewness while the median would stay put as it is resistant to outliers. Histogram J however, shows the mean being greater than the median as it is being pulled to the right.
-
The resulting histogram of the combination of the two groups of students who walk to school and time it takes for school would be normally distributed.
-

-
Name of test: 2 sample t-test. We want to test a claim about the difference between mu1 (the true mean travel time it takes to travel to school for 50 students) and mu2 (the true mean travel time it takes to travel to school for 50 students.
Teacher Feedback
Your answer for part 1 is very good - you pick the correct histogram and give a clear reason for doing so (and clearly explain why it WOULDN’T be the other histogram). Full credit. For part 2, you say “normal,” which implies a unimodal graph with a peak in the center of the distribution. That won’t be the case here: if you look carefully at the x-axis for each distribution the peaks will end up separated (one around 8 minutes and the other around 40 minutes), whereas the center of the combined number line (around 24 minutes) has very few observations. Thus, bimodal is the description we’d be looking for here.
For part 3, your work is good - you’ve checked conditions, cited the use of the CLT, and correctly calculated the parameters of the sampling distribution. One thing - you never mention the shape (just saying “normality” as a condition isn’t equivalent to saying “the sampling distribution would be approximately normal.”). Therefore, you’d get partial instead of full credit. Advice - after citing the CLT, state that this means the sampling distribution is approximately normal.
Finally, in part 4, you’d also receive partial credit, if any. A 2-sample t-test is the correct test. However, you do not write fully correct statements for Ho and Ha. Your Ho would be that the mean travel times for the two groups are the same; Ha would be that the mean travel times for the groups are different. You only mention the ‘difference’ and do not cite it as the alternative hypothesis. Additionally, you define mu1 and mu2 as representing 50 students - but they would represent ALL students and ALL teachers at the school (since “mu” is a population parameter). I’m working on the assumption that you meant for one of your parameters to mention teachers. Be careful when typing/writing responses!
Student Response 2
- If the mean time is lower than the median time, that means the distribution is skewed to the left and would be appropriate for the distribution of histogram K. this is because when the distribution is skewed, the mean is pulled to the tail of the distribution and is either larger than or smaller than the median. In this case, for the mean to be smaller, the tail should be to the left.
- The distribution of both histograms J and k combined will have a bimodal shape and the peaks would be around 0-8 and 40-48.
- For a random sample of 36 staff members, the sampling distribution will follow a approximately normal distribution and have a mean of 25 minutes and a standard deviation of 18/ (square. root of 36) = 3 minutes. Because the sample size of 36 is larger than 30, it meets the CLT condition and can be assumed as approximately normal.
- The test would be two sample t test for the difference of means1. Ho : MUs - MUt =01. Ha : MUs - MUt does not equal 0.1. MUs represents the true mean travel time for students and MUt represents the true mean travel time for teachers/ staff
Teacher Feedback
All of your answers are correct and give appropriate reasoning. A tip for part (a): when asked to make a choice in AP stats, you should explain not only why your choice is correct, but why the other option is incorrect. You could add “since histogram J is right skewed, it does not match the description” or something similar. All of your other answers are thorough, in context, and show strong understanding. Well done!
Student Response 3
- If the mean is less than the median that means the data is skewed to the left, because the mean is affected by smaller values bringing it down. Histogram K appears skewed to the left, so it must represent the group of students who walk to school.
- The distribution can be described as bimodal.
- The shape of the histogram is approximately normal, because 36 is greater than 30, which satisfies the central limit theorem. The center is the mean, 25 minutes. The spread is the standard error, 18/sqrt36, or 3.
- Two sample means t test1. H0: mu1 = mu21. HA: mu1 is not equal to mu21. where mu1 is the mean travel time for students and mu2 is the mean travel time for teachers. Conditions:
- The students and teachers were randomly selected.
- 50 and 40 are both greater than 30, which satisfies the Central Limit Theorem, indicating that the distributions are approx. normal.
- There are at least 501 students and 401 teachers, so the 10% condition for independence is satisfied.
Teacher Feedback
Nice job! One small thing: for #2, you’re correct in calling the shape “bimodal,” but the rubric for a similar question only gave full credit for identifying where the clusters/peaks were centered. So you’d get partial credit. Full credit: “bimodal, with peaks at around 8 minutes and 40 minutes”

© 2024 Fiveable Inc. All rights reserved.