Browse By Unit
5.1 Using the Mean Value Theorem
In the previous unit, we learned all about applying derivatives to different real-world contexts. What else are derivatives useful for? Turns out, we can also use derivatives to determine and analyze the behaviors of functions! 👀
📈 Mean Value Theorem
The Mean Value Theorem states that if a function f is continuous over the interval and differentiable over the interval , then there exists a point within that open interval where the instantaneous rate of change of the function at equals the average rate of change of the function over the interval .
In other words, if a function f is continuous over the interval and differentiable over the interval , there exists some on such that .
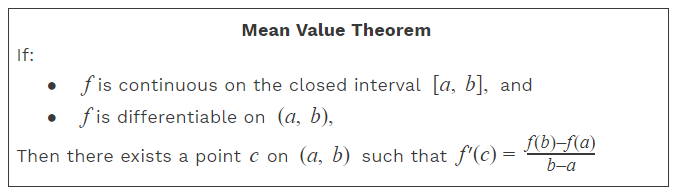
Image Courtesy of Sumi Vora and Ethan Bilderbeek
Yet another way to phrase this theorem is that if the stated conditions of continuity and differentiability are satisfied, there is a point where the slope of the tangent line is equivalent to the slope of the secant line between and .
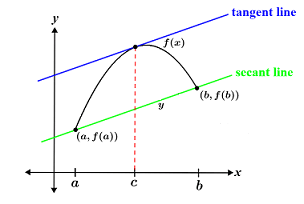
Image Courtesy of Math.net
Remember from Unit 1, to be continuous over means that there are no holes, asymptotes, or jump discontinuities between points a and b. Because the interval contains closed brackets, the graph must also be continuous at points and .
Additionally, if we recall from previous guides, to be differentiable over means that the function is continuous over the interval and that for any point over the interval, exists.
✏️ Mean Value Theorem: Walkthrough
We can use the Mean Value Theorem to justify conclusions about functions by applying it over an interval. For example:
Let be a differentiable function. The table gives its selected values:
Can we use the Mean Value Theorem to say the equation has a solution where ?
Since it is given that is differentiable, we can apply the Mean Value Theorem (MVT) on the interval . This is what we should write out!
Since is continuous and differentiable on , MVT can be applied. The MVT states that there exists a on such that
so MVT cannot be used to say that has a solution.
📝 Mean Value Theorem: Practice Problems
Now, it’s time for you to do some practice on your own! 🍀
❓ Mean Value Theorem: Practice
Question 1: Mean Value Theorem
Let and let be the number that satisfies the Mean Value Theorem for on the interval .
What is
Question 2: Mean Value Theorem
Let be a differentiable function. The table gives its selected values:
Can we use the Mean Value Theorem to say the equation has a solution where ?
✅ Mean Value Theorem: Answers and Solutions
Question 1: Mean Value Theorem
Since is a polynomial, is continuous on and differentiable on . Therefore, the Mean Value Theorem can be applied. By the Mean Value Theorem, there exists a on such that…
To find , we need to differentiate and find such that
By the quadratic formula, we have .
Since only is in the interval , Great work! Make sure you remember to check if the value(s) you get are in the given interval.
Question 2: Mean Value Theorem
Since it is given that is differentiable, we can apply the Mean Value Theorem (MVT) on the interval .
The MVT states that there exists a on such that so MVT cannot be used to say that has a solution.
💫 Closing
The Mean Value Theorem states that for any continuous function on a closed interval, there exists a value c in the interval such that the value of the derivative of the function at c is equal to the average rate of change of the function over the interval. By using this theorem, we can find the mean value of a function on a given interval, which can provide useful information about the behavior of the function. 🧐
<< Hide Menu
5.1 Using the Mean Value Theorem
In the previous unit, we learned all about applying derivatives to different real-world contexts. What else are derivatives useful for? Turns out, we can also use derivatives to determine and analyze the behaviors of functions! 👀
📈 Mean Value Theorem
The Mean Value Theorem states that if a function f is continuous over the interval and differentiable over the interval , then there exists a point within that open interval where the instantaneous rate of change of the function at equals the average rate of change of the function over the interval .
In other words, if a function f is continuous over the interval and differentiable over the interval , there exists some on such that .

Image Courtesy of Sumi Vora and Ethan Bilderbeek
Yet another way to phrase this theorem is that if the stated conditions of continuity and differentiability are satisfied, there is a point where the slope of the tangent line is equivalent to the slope of the secant line between and .

Image Courtesy of Math.net
Remember from Unit 1, to be continuous over means that there are no holes, asymptotes, or jump discontinuities between points a and b. Because the interval contains closed brackets, the graph must also be continuous at points and .
Additionally, if we recall from previous guides, to be differentiable over means that the function is continuous over the interval and that for any point over the interval, exists.
✏️ Mean Value Theorem: Walkthrough
We can use the Mean Value Theorem to justify conclusions about functions by applying it over an interval. For example:
Let be a differentiable function. The table gives its selected values:
Can we use the Mean Value Theorem to say the equation has a solution where ?
Since it is given that is differentiable, we can apply the Mean Value Theorem (MVT) on the interval . This is what we should write out!
Since is continuous and differentiable on , MVT can be applied. The MVT states that there exists a on such that
so MVT cannot be used to say that has a solution.
📝 Mean Value Theorem: Practice Problems
Now, it’s time for you to do some practice on your own! 🍀
❓ Mean Value Theorem: Practice
Question 1: Mean Value Theorem
Let and let be the number that satisfies the Mean Value Theorem for on the interval .
What is
Question 2: Mean Value Theorem
Let be a differentiable function. The table gives its selected values:
Can we use the Mean Value Theorem to say the equation has a solution where ?
✅ Mean Value Theorem: Answers and Solutions
Question 1: Mean Value Theorem
Since is a polynomial, is continuous on and differentiable on . Therefore, the Mean Value Theorem can be applied. By the Mean Value Theorem, there exists a on such that…
To find , we need to differentiate and find such that
By the quadratic formula, we have .
Since only is in the interval , Great work! Make sure you remember to check if the value(s) you get are in the given interval.
Question 2: Mean Value Theorem
Since it is given that is differentiable, we can apply the Mean Value Theorem (MVT) on the interval .
The MVT states that there exists a on such that so MVT cannot be used to say that has a solution.
💫 Closing
The Mean Value Theorem states that for any continuous function on a closed interval, there exists a value c in the interval such that the value of the derivative of the function at c is equal to the average rate of change of the function over the interval. By using this theorem, we can find the mean value of a function on a given interval, which can provide useful information about the behavior of the function. 🧐

© 2024 Fiveable Inc. All rights reserved.